This manuscript has been
published in Monthly
Weather Review, the December 1999 issue, Vol. 127,
pp. 2709-2732; Corrigendum, 128, 1573.
A Corrigendum for Schultz and Schumacher (1999)
has been published in the May 2000 issue of Monthly Weather
Review. The correct way to reference our paper is:
Schultz, D. M., and P. N. Schumacher, 1999: The use and misuse of
conditional symmetric instability. Mon. Wea. Rev., 127,
2709-2732; Corrigendum, 128, 1573.
[AMS]
[Corrigendum]
[PDF]
[PDF
of Corrigendum]
[HTML]
© Copyright
1999, American Meteorological Society
More information about Conditional Symmetric
Instability can be found at the CSI Homepage.
The Use and Misuse of
Conditional Symmetric Instability
Philip N. Schumacher
NOAA/National Weather Service, Sioux Falls, South Dakota
For Publication in
REVIEW
Monthly Weather Review
Submitted June 1998, Revised November 1998 and December 1998
Accepted 14 December 1998
Corresponding author address:
Dr. David M. Schultz
NOAA/National Severe Storms Laboratory
1313 Halley Circle
Norman, OK 73069
E-mail: david.schultz@noaa.gov
Abstract
A commonly employed explanation for single- and multiple-banded clouds
and precipitation in the extratropics is slantwise convection due to
the release of moist symmetric instability (MSI), of which one type is
conditional symmetric instability (CSI). This article presents a
review of CSI with the intent of synthesizing the results from
previous observational, theoretical, and modeling studies. It is our
contention that CSI as a diagnostic tool to assess slantwise
convection has been, and continues to be, misused and overused.
Drawing parallels to an ingredients-based methodology for forecasting
deep, moist convection that requires the simultaneous presence of
instability, moisture, and lift, some of the misapplications of CSI
can be clarified. Many of these pitfalls have been noted by earlier
authors, but are, nevertheless, often understated, misinterpreted, or
neglected by later researchers and forecasters.
Topics include the evaluation of the potential for slantwise
convection, the relationship between frontogenesis and MSI, the
coexistence of moist gravitational instability and MSI, the nature of
banding associated with slantwise convection, and the diagnosis of
slantwise convection using mesoscale numerical models. The review
concludes with suggested directions for future observational,
theoretical, and diagnostic investigation.
1 Introduction
Single- and multiple-banded [hereafter, banded (Huschke 1959,
57)] clouds and precipitation are commonly observed in association
with frontal zones in extratropical cyclones (e.g., Hobbs 1978; Houze
1993, section 11.4). Proposed explanations for these bands (e.g.,
Parsons and Hobbs 1983; Emanuel 1990) include frontogenesis,
boundary-layer instabilities, ducted gravity waves, Kelvin-Helmholtz
instability, and moist slantwise convection due to the release of
conditional symmetric instability (CSI; symbols and acronyms appearing
in this article are listed in the appendix). A precise definition for
CSI, a type of moist symmetric instability (MSI), is given in
section 3b. Indeed, some observational studies over
extended periods of time show the presence of MSI in association with
banded precipitating baroclinic systems to be rather common (e.g.,
Bennetts and Sharp 1982; Seltzer et al. 1985; Byrd 1989; Reuter and
Yau 1990, 1993; Reuter and Aktary 1993, 1995).
Although we do not deny the likely existence of slantwise convection
or the possible involvement of MSI in some precipitating systems in
the atmosphere, it is our contention that CSI is frequently misused
and overused as a diagnostic tool, a point also noted by Wiesmueller
and Zubrick (1998, 86).
We believe the following four reasons are responsible, in part, for
the present situation.
(1) The theory of CSI has certain limitations and caveats that are
discussed by earlier authors, but often are understated,
misinterpreted, or neglected by later researchers and forecasters who
rely on CSI as an explanation for banded precipitation. As the
concept of CSI had grown in popularity and usage over time
(Fig. 1), these qualifications are often omitted as
second-generation references are cited at an increasing rate, instead
of the older, but perhaps more correct, references.
(2) With the advent of satellite imagery and Doppler radar with higher
resolution than was available previously, banded cloud and
precipitation features are observed frequently and demand explanation.
These bands possess many of the characteristics expected from CSI
theory (Emanuel 1983c, 233) and they tend to be present in regions
where the criteria for CSI are satisfied. Hence, CSI typically is
concluded to have played a role in their formation, at the expense of
other, perhaps more plausible, mechanisms.
(3) Due to the availability of gridded numerical-model data and the
popularization of CSI diagnostics by forecasters (e.g., Dunn 1988;
Lussky 1989; Grumm and Nicosia 1997; Wiesmueller and Zubrick 1998),
evaluation of CSI locally at universities, laboratories, and forecast
offices is becoming more common.
With the relative computational ease of CSI-related parameters and the
increasing emphasis on short-term quantitative precipitation
forecasting, CSI frequently becomes a default explanation for banded
precipitation.
(4) Whereas idealized modeling studies of flows possessing CSI have
been performed, the applicability of those results to observations may
be questionable. Consequently, such theoretical and numerical results
often are unappreciated by observational meteorologists. Similarly,
observational results usually are not described from a theoretical or
numerical-modeling framework. This leads to an ever-increasing rift
between theory and observations of CSI.
Thus, for these four reasons, CSI is commonly observed, yet, often
misinterpreted and misunderstood.
The purpose of this article is twofold: to attempt to limit further
misuse of the CSI paradigm by researchers and forecasters alike by
highlighting common pitfalls, and to encourage future research
explorations that are directed at the deficiencies in our
understanding of MSI and slantwise convection.
The remainder of this article is as follows. In
section 2, we develop parallels between an
ingredients-based methodology for forecasting moist gravitational
convection (also known as buoyant or upright convection) and slantwise
convection. These three ingredients of instability, moisture, and
lift are explored further in this article: section 3
reviews the calculation of symmetric instability and
section 4 discusses moisture and lift, specifically the
relationship between MSI and frontogenesis. In section 5,
the implications for the coexistence of instabilities to both moist
gravitational and slantwise convections are examined, including a
discussion of the structure, evolution, dynamics, and possible
electrification of weather systems that might result from such
scenarios. Section 6 investigates the nature of cloud and
precipitation banding in relation to MSI and section 7
addresses the diagnosis of slantwise convection using mesoscale
numerical-model output. Finally, section 8 consists of a
summary of main points, directions for future research, and a
concluding discussion.
2 An ingredients-based methodology for slantwise convection
Throughout this article, we wish to differentiate between free
convection and forced convection as motions in the atmosphere
that are associated with the presence and absence of instability,
respectively (Huschke 1959, 133-134; Emanuel 1980, 192-193). Unless
otherwise specified, we use the generic term convection to imply
free convection (gravitational or symmetric).
In order to clarify some of the confusion surrounding the concepts of
CSI and slantwise convection, we find it useful to demonstrate
parallels with the more familiar concepts of moist gravitational
instability and convection. An exploration of these parallels begins
with an ingredients-based methodology for forecasting deep, moist
convection (McNulty 1978, 664; Doswell 1987, 7; Johns and Doswell
1992, 589-590; McNulty 1995, 189-191; Doswell et al. 1996, 563).
This methodology asserts that three ingredients are required to
produce deep, moist convection: instability, moisture, and lift
(Fig. 2). As Doswell (1987, 7) notes, ``Remove any one
of these and there well may be some important weather phenomena, but
the process is no longer deep, moist convection.''
For the purposes of this article, we adopt the same triad of
ingredients from moist gravitational convection (instability,
moisture, and lift) for the production of moist slantwise convection,
where the requisite instability becomes MSI, rather than moist
gravitational instability.
Although some previous studies use the ingredients-based methodology
implicitly,
our interpretation of some of the other previous literature is that
CSI is treated sometimes as an instability and sometimes as a forcing
mechanism for ascent. An example is illustrated by those who wish to
separate the effect of CSI from that due to frontogenesis, when in
fact these two processes often cannot be considered independently (see
section 4). The ingredients-based methodology firmly labels
CSI as the instability, clearly separate from the lifting mechanism.
Applying the ingredients-based methodology to slantwise convection
indicates that the existence of CSI alone is not sufficient to
initiate slantwise convection. Employing CSI in this manner is
tantamount to saying that the occurrence of a severe thunderstorm is
due solely to the presence of conditional instability to moist
gravitational convection! Since slantwise convection is the process
by which the instability is released, it follows that the terms
moist slantwise convection and CSI do not have the same
meaning, contrary to their implied equivalence by some authors (e.g.,
Wolfsberg et al. 1986, p. 1552; Moore and Blakley 1988, p. 2167;
Howard and Tollerud 1988, 170; Lemaître and Testud 1988, 261;
Bennetts et al. 1988, 368; Emanuel 1990, 473; Reuter and Yau 1993,
375; Lagouvardos et al. 1993, p. 1293; Grumm and Nicosia 1997, 10;
Wiesmueller and Zubrick 1998, 85; Koch et al. 1998, p. 2040).
In contrast, Thorpe and Rotunno (1989, 1293-1294) have suggested that
``slantwise convection'' is not appropriate terminology to describe
the circulations that release CSI. Taking the fluid dynamicists'
definition of convection to be a circulation that results in positive
heat flux, Thorpe and Rotunno (1989) note that the heat flux with CSI
circulations can be negative in certain cases (e.g., Miller 1984),
and, hence, these circulations formally should not be considered
``convection.'' These thoughts on terminology, however, are not
shared by all in the atmospheric-science community. For example, it
has been argued that the equations for symmetric instability are
isomorphic to both inertial instability and gravitational instability
(e.g., Emanuel 1983b, 2016-2017; Xu and Clark 1985; Emanuel 1994,
394-395). Therefore, the release of each instability individually
can be considered a form of ``convection.'' In agreement with this
latter argument, we choose the term slantwise convection to represent
the release of MSI.
It should be noted that there are limitations to this analogy
between slantwise and gravitational convection. As noted by Emanuel
(1990, 473), ``The fact that precipitation falls vertically and that
turbulence may not be isotropic may serve to make the physics of
slantwise convection quite different from that of upright convection,
though this has hardly been explored.'' Further discussion of
turbulence as it relates to slantwise convection can be found in
Emanuel (1994, 406).
As is shown throughout this article, the occurrence of slantwise
convection depends upon all three ingredients and all must be present
in order to justify any claims of slantwise convection. Next, in
section 3, we examine how symmetric instability is
evaluated. Moisture and lift are discussed in section 4.
3 Assessing symmetric instability
The derivation of dry and moist symmetric instability is not presented
here. Interested readers are referred to the following sources for
further information. Early work on symmetric instability by
Helmholtz, Rayleigh, Solberg, Bjerknes and Godske, Kleinschmidt, Fjø
rtoft, Sawyer, Eliassen, Kuo, Ooyama, Stone, McIntyre, and others is
reviewed by van Mieghem (1951), Emanuel (1979), Xu (1986b), and
Shutts (1990). The basics of dry symmetric instability are presented
in Lilly (1986), Holton (1992, section 9.3), and Bluestein (1993,
339-350). Derivations of MSI can be found in the pioneering works of
Bennetts and Hoskins (1979) and Emanuel (1983a,b), or the excellent
textbook presentations of Bluestein (1993, section 3.5.2), Houze
(1993, section 2.9.1), and Emanuel (1994, chapter 12).
3a Dry symmetric instability
Dry symmetric instability is a generalization of both inertial
instability and dry gravitational instability. The term
symmetric refers to a basic state and perturbations that are
independent of a horizontal direction, here assumed to be parallel to
the y axis. Table 1 compares
gravitational, symmetric, and inertial instabilities, illustrating the
parallelism in their definitions and measures. As noted by Jones and
Thorpe (1992, 231), ``at the present time, symmetric instability
theory is applicable only to two-dimensional flow.'' Therefore, when
applying this theory to observational data, it must be remembered that
a three-dimensional perturbation on such a flow may not necessarily
release the instability, an issue dealt with in more detail by Jones
and Thorpe (1992) and Gu et al. (1998).
In the Northern Hemisphere (assumed throughout this article), the
condition for inviscid, inertial instability is
¶Mg /¶x < 0, where Mg = vg+fx is the geostrophic absolute
momentum (also known as geostrophic pseudoangular momentum) of
a geostrophically balanced mean state, vg is the geostrophic wind
in the direction perpendicular to the temperature gradient (hereafter,
the along-front direction, although strictly we are considering a
baroclinic zone, not necessarily a front), f is the Coriolis
parameter, and x is the cross-front distance, increasing toward
warmer air. This condition is equivalent to the geostrophic absolute
vorticity being negative. Similarly, the condition for inviscid, dry
gravitational instability (what is typically referred to as dry
absolute instability by meteorologists) is that the hydrostatically
balanced mean-state potential temperature [`(q)] decreases
with height z (i.e., ¶[`(q)] / ¶z < 0).
Whereas a parcel may be inertially stable to horizontal (constant z)
displacements (¶Mg / ¶x > 0) and gravitationally
stable to vertical displacements (
¶[`(q)] / ¶z > 0) separately, it may be unstable with respect to slantwise
displacements by dry symmetric instability. The condition for dry
symmetric instability is that Mg surfaces slope less steeply than
[`(q)] surfaces (hereafter called the
Mg-[`(q)] relationship). Dry symmetric instability can
be viewed as either dry gravitational instability on a Mg surface
(i.e., ¶[`(q)] / ¶z |Mg < 0) or inertial
instability on an isentropic surface (i.e.,
¶Mg / ¶x|[`(q)] < 0). Therefore, any slantwise displacement that
occurs at an angle between the slopes of the Mg and [`(q)]
surfaces will release the symmetric instability.
In the strictest sense, symmetric instability should be determined
relative to these hydrostatically and dynamically (in this case,
geostrophically) balanced mean states. [See Persson and Warner (1995,
3457-3458) and Xu (1992, 632) for further discussion of this point.]
Since the atmosphere is hydrostatic to a very good approximation,
using the environmental potential temperature q instead of
[`(q)] probably introduces minimal error. In general, though,
the atmosphere is not geostrophic and may, in fact, exhibit large
departures from geostrophy in regions susceptible to symmetric
instability (e.g., Seltzer et al. 1985, section 3c; Wolfsberg et
al. 1986, p. 1553; Knight and Hobbs 1988, 926; Byrd 1989, 1128-1129).
In observational studies that assess symmetric instability from
limited observations such as single soundings, the geostrophic wind is
often approximated by the total wind and stated explicitly as such
(e.g, Emanuel 1983b, 1988; Byrd 1989; Reuter and Aktary 1995). An
additional complication occurs with mesoscale-model data in which the
geostrophic wind (derived from the geopotential height) is often much
noisier than the total wind (Shutts 1990, p. 2747), although methods
have been developed to construct well-behaved fields from
numerical-model data (e.g., Barnes et al. 1996). In time, more
researchers and forecasters are approximating Mg by M (i.e.,
M = v+fx, where M is the absolute momentum calculated from the total
along-front wind v), interchanging the total wind v for the
geostrophic wind vg, even when the geostrophic wind is readily
available (e.g., Martin et al. 1992; Leach and Kong 1998; Chen et
al. 1998).
The use of M instead of Mg is inconsistent with the theory for
symmetric instability discussed above. If significant flow curvature
is present (especially changes in curvature in the vertical),
the observed along-front wind v is better approximated by the
along-front gradient wind vgr rather than the along-front
geostrophic wind vg. Sanders (1986, section 4b) derives the
condition for symmetric instability relative to a basic state in
gradient-wind balance and presents nomograms for its use. That
condition is
-3[`(v)]gr( 1+[`(v)]gr/fr)/r < 0, where [`(v)]gr is the basic-state along-front
gradient-wind speed and r is the distance along the radius of
curvature.
By using the observed wind to compute M, rather than the geostrophic
wind, situations with strong cyclonic shear (near-horizontal M
surfaces) may be assessed to be more ``inertially" stable to ascent
along a [`(q)] surface than they really are, since the
computed M surface includes the additional balance provided by the
centrifugal force. In addition, in areas with orography, large
pressure changes, or intense frontogenesis, significant ageostrophy in
the observed lower-tropospheric wind can occur. In those cases, the
geostrophic wind may be unidirectional but the observed wind may have
significant curvature (and not be nearly two-dimensional). Extensions
of this theory to these situations of questionable two-dimensionality
are considered in section 3c.
Therefore, to assess the condition for dry symmetric instability,
replacing the hydrostatically balanced basic state [`(q)] by
q is generally a valid approximation.
Hereafter, throughout this article, we approximate [`(q)] by
q.
In contrast, replacing the geostrophically balanced basic state Mg
by M may be a poor approximation, but this subject has not been
addressed extensively in the literature. For example, in some
situations, while the observed wind may not be similar to the
geostrophic wind, their vertical gradients, the factor important in
calculating M/Mg, may be more similar. Until further
investigation is performed to evaluate the importance of this
assumption, we recommend use of the geostrophic wind.
A final point is that separating the data (observations or
model-derived) into basic state and perturbation is a complicated
issue in its own right (i.e., the so-called partitioning problem). In
practice, the geostrophic wind is usually computed directly from the
total pressure or height field. This implicitly assumes that the
perturbation pressure caused by the unbalanced part of the flow (such
as gravitational convection, MSI circulations, and gravity waves) is
negligible, an assumption that may not be valid after these
perturbations are already present. In this case, how to partition the
total flow is not a trivial matter, especially when CSI is evaluated
in three-dimensional space with an extended criterion applied to
geostrophic flow (e.g., Shutts and Cullen 1987) or other nonlinearly
balanced flow (e.g., Xu 1994, appendix C). How, and even whether, to
perform the partition and what balanced system to use (e.g.,
geostrophy, gradient-wind balance, semigeostrophy, or higher-accuracy
dynamical system) remain difficult questions. Even if complete data
are available for performing a partition in space and time, the
partition may not be precisely or uniquely determined. Unfortunately,
our understanding of these issues is still very limited and may
profoundly affect our assessment of symmetric stability from
atmospheric observations. If, however, such complicated partitions
are deemed necessary in order to assess MSI rigorously, they are
unlikely to become operationally feasible.
3b Moist symmetric instability: Conditional symmetric
instability versus potential symmetric instability
The addition of moisture complicates the theory of symmetric
instability considerably.
For moist gravitational convection, conditional instability (CI)
occurs locally at each height along a vertical sounding where the
environmental lapse rate lies between the moist and dry adiabatic
lapse rates, or, equivalently, qe*, the saturation
equivalent potential temperature1, decreases with height (i.e.,
¶qe* /¶z < 0).
Similarly, for slantwise convection, CSI occurs locally at each height
where the environmental lapse rate along a Mg surface lies between
the moist and dry adiabatic lapse rates (i.e., is conditionally
unstable along a Mg surface), or
¶qe* / ¶z|Mg < 0.
The instability is said to be conditional because saturation
must be present locally in order for air parcels to be unstable (i.e.,
possess parcel buoyancy).2
Contrast these definitions for conditional instabilities to those for
potential instabilities.
The difference between CI and PI has been discussed by, for example,
Wallace and Hobbs (1977, section 2.7.3), Rogers and Yau (1989, 35),
and Emanuel (1994, 185).
As in moist gravitational convection where the potential instability
(PI; also known as convective instability) of a layer along a vertical
sounding can be defined (¶qe / ¶z < 0), it is
possible to assess layer potential symmetric instability (PSI) along a
Mg surface: ¶qe / ¶z |Mg < 0.
The instability is said to be potential because the potentially
unstable layer first must undergo a finite vertical displacement to
reach saturation and create the instability; release of the
instability may then result, given sufficient forcing for ascent.
It can be shown that the criterion for PSI is equivalent to the
Mg-qe relationship. Therefore, the commonly employed
method of assessment for CSI in the literature, the Mg-qe
relationship, is really a measure of PSI, not CSI, if we follow the
definitions from moist gravitational convection.
Strictly, the saturation equivalent potential temperature qe*
should be used to assess CSI, as noted by several authors (Emanuel
1988; Reuter and Yau 1990, 449; Thorpe and Clough 1991; Houze 1993).
There can be a substantial difference between qe* and
qe, as illustrated by Fig. 3, a cross section
across an equatorward-moving lower-tropospheric cold front over the
northern United States. Although the air above the frontal surface is
conditionally unstable since qe* decreases with height
(Fig. 3a), it is potentially stable since qe
increases with height (Fig. 3b). This case illustrates
the importance of recognizing possible differences between
qe* and qe and its relationship to assessing MSI.
The wet-bulb potential temperature qw is also a conserved
variable under dry and pseudoadiabatic processes and may be
equivalently employed for qe in diagnosing PSI. At
saturation, the vertical gradients of qe, qe*, and
qw are equivalent, therefore, CI and PI, as well as CSI and
PSI, are equivalent. More will be said about determining saturation
using observational data and mesoscale models in sections 4
and 7.
It is surprising to note that this misnomer of CSI has deep roots and
likely arises from later authors' misinterpretation of the original
work. Bennetts and Hoskins (1979, 949-951) define CSI for a
``two-dimensional flow in an atmosphere which is assumed to be
saturated everywhere'' as a natural extension of CI, which they define
as the wet-bulb potential temperature decreasing with height. As
mentioned previously, CI and PI are equivalent concepts only when the
atmosphere is saturated. Thus, many later researchers and forecasters
continue to use qe/qw and the term CSI, but may not
realize the assumption of saturation, explicitly stated by Bennetts
and Hoskins (1979). Also, meteorologists' familiarity with qe
as a tracer of dry and irreversible moist processes and the ability to
compute qe readily in meteorological analysis packages
probably facilitate the use of qe over qe* in
assessing MSI.
Despite the fact that several authors define and use CSI correctly in
their own work (e.g., Reuter and Yau 1990, 449; Houze 1993, 54-56;
Emanuel 1994, 410), this misuse of the term CSI continues and now
pervades the literature. We would like to suggest that, in the
future, we, as a meteorological community, use the term CSI only when
employing qe* and use the term PSI only when employing
qe. We adopt this terminology throughout the rest of the
article. In addition, we use the term MSI when the method of
assessment is unimportant or is not specified.
The question naturally arises as to whether it is more appropriate to
calculate CSI or PSI for assessing the possibility of moist slantwise
convection in the atmosphere. Drawing an analogy to moist
gravitational convection may help provide some insight. The necessary
condition for moist gravitational convection is that a rising air
parcel be saturated and the lapse rate be greater than the moist
adiabatic lapse rate (CI), so that positive buoyancy exists. Meeting
the lapse-rate requirement for PI or PSI is only a necessary condition
for release of the instability; saturation must also occur.
Consequently, the presence of PI is not necessary for moist
gravitational convection to occur in the atmosphere (e.g., Emanuel
1994, 185).
Therefore, throughout the rest of this article, we use the term CSI,
computed with the proper thermodynamic variable qe*, as the
appropriate measure of the susceptibility of the atmosphere to
slantwise convection. Thus, many previous studies may not determine
the true potential for slantwise convection because of their use of
qe and PSI, rather than qe* and CSI.
3c The validity of the Mg-qe relationship and
moist geostrophic potential vorticity
Certain assumptions are necessary in order to develop the
Mg-qe* relationship for the identification of CSI: (1)
the geostrophic wind is constant in the along-front direction, (2) the
cross section for evaluating the Mg-qe* relationship is
perpendicular to the vertical shear of the geostrophic wind (or,
equivalently, the thermal wind or isotherms), and (3) the ageostrophic
wind (for example, due to flow curvature) is small so that the
geostrophic wind is a reasonable approximation to a basic state.
Discrepancies in interpreting the existence of CSI in cross sections
can result, therefore, because one or more of the above criteria is
not strictly met. Further discussion of this point, and the extension
to three dimensions of the Mg-qe relationship for
assessing PSI, is found in Shutts and Cullen (1987) and Shutts (1990).
It can be shown that extending the Mg-q relationship for
dry symmetric instability to three dimensions is equivalent to
computing geostrophic potential vorticity PVg (e.g., Hoskins 1974),
where PVg = ghg ·Ñq, g is gravity, hg
is the three-dimensional geostrophic absolute vorticity vector, and
Ñ is the gradient operator in x, y, and z coordinates.
When PVg is negative (and inertial and dry gravitational
instabilities are absent), dry symmetric instability is present.
Likewise, the three-dimensional form of the Mg-qe*
relationship for CSI is equivalent to negative saturated geostrophic
potential vorticity MPVg* (also known as the saturated equivalent
geostrophic potential vorticity, MPV
g* = g hg ·Ñqe*), when inertial and conditional instabilities are absent.
Also, the three-dimensional form of the Mg-qe relationship
for PSI is equivalent to negative moist geostrophic potential
vorticity MPVg = g hg ·Ñqe, when inertial and
potential instabilities are absent.
Therefore, assessing CSI using the three-dimensional form of MPVg*
does not require strict adherence to the same assumptions as using the
Mg-qe* relationship in cross-section form.
Owing to the potential confounds with assessing Mg-qe*
relationships in cross sections, a more reliable assessment of CSI is
obtained by employing MPVg* or another equivalent
three-dimensional parameter.
On the other hand, use of MPVg/MPVg* as a diagnostic tool does
not differentiate between regions of PI/CI and PSI/CSI (e.g., McCann
1995; Wiesmueller and Zubrick 1998, 94-95). Likewise, regions of
inertial instability and convective stability may also indicate
negative MPVg/MPVg*. Therefore, MSI diagnostics always should
be employed in conjunction with tests for moist gravitational and
inertial instabilities. Further discussion of this point is found in
section 5.
Other three-dimensional parameters that are used to assess MSI include
a growth-rate parameter (e.g., Bennetts and Hoskins 1979, 952;
Bennetts and Sharp 1982, 596), a moist Richardson number (e.g.,
Sanders and Bosart 1985, equation 4; Seltzer et al. 1985), and a moist
symmetric-stability parameter (e.g., Jascourt et al. 1988, 181).
From the three-dimensional form of MPVg, Moore and Lambert (1993)
derive a two-dimensional form of MPVg [their equation (2)] and use
it to assess PSI in their cross sections. Consequently, their results
depend upon the orientation of the cross section as in the
Mg-qe relationship (i.e., the full potential of MPVg as
a three-dimensional parameter for diagnosis is not being utilized).
Thus, even in cross sections, application of the full
three-dimensional form of MPVg is essential for an accurate
assessment of PSI. Unfortunately, employing the two-dimensional form
of MPVg has been increasing in popularity (e.g., Weisman 1996;
Wiesmueller and Zubrick 1998).
3d Slantwise convective available potential energy
Analogous to convective available potential energy (CAPE) for
gravitational convection (e.g., Emanuel 1994, 169-172), slantwise
convective available potential energy (SCAPE) can be defined (e.g.,
Emanuel 1983b, sections 2 and 3; Shutts 1990; Emanuel 1994, 407-409).
These parameters measure the amount of positive area on a
thermodynamic diagram and represent the maximum kinetic energy
possible from adiabatic, inviscid parcel theory. In the case of CAPE,
it is the maximum kinetic energy of a vertical updraft; in the case of
SCAPE, it is the maximum kinetic energy of an updraft along a Mg
surface,3 consisting of both
horizontal and vertical motions, contrary to Black et al. [1994, their
eq. (11); although their later assumption that
¶qe /¶z = 0 mitigates this discrepancy] and McCann (1996, 122) who
use SCAPE as a measure of vertical motion only.
The use of SCAPE as a measure of the potential for slantwise
convection has not been particularly common in the literature, despite
the similarity to its more popular relative CAPE. Byrd (1989) finds
that values of SCAPE were of limited value in determining the degree
of banding within precipitating regions over the southern Plains,
whereas Shutts (1990) shows that values of SCAPE derived from
mesoscale numerical-model output are somewhat related to explosive
cyclogenesis over the North Atlantic Ocean. Most other examples in
the literature involve the computation of SCAPE for individual cases
and therefore cannot be placed within a broader context relative to
other events. The lack of studies on SCAPE makes its interpretation
as a diagnostic tool uncertain, but can also be viewed as SCAPE not
being a particularly useful parameter. These issues are compounded by
(1) the difficulty in computing SCAPE from upper-air observations, (2)
the relatively small values of SCAPE typically observed in regions of
active slantwise convection (see section 4), and (3) the
small values of SCAPE in comparison to CAPE, when CAPE is zero or
small. These difficulties make further investigation of the utility
of SCAPE difficult (McCarthy 1996), probably limiting more widespread
usage.
3e Neglected effects
It is important to note that the concept of MSI, like gravitational
instability, is based upon parcel theory. Therefore, the caveats and
limitations with using parcel theory for gravitational convection also
apply to using parcel theory for slantwise convection. [For further
discussion on parcel theory as applied to symmetric instability, see
Thorpe et al. (1989).] In particular, assumptions about mixing and
microphysical processes strongly affect computations of parcel
buoyancy.
One example is the weight of condensed water on buoyancy (also known
as water loading). Reuter and Yau (1993), following the methodology
of Xu and Emanuel (1989), find that soundings exhibiting MSI are
actually neutral or stable to moist slantwise ascent when the effects
of water loading on the virtual temperature are included. To include
the effects of water loading, however, the assumption must be made
that all condensed water remains with the parcel, an assumption that
obviously fails in precipitating clouds and may fail in other
situations as well (Xu and Emanuel 1989, section 5). Williams and
Renno (1993) find that, if water loading is included, then logically
this water must be frozen, if it reaches sufficient height. They find
that the additional positive buoyancy due to release of this latent
heat of fusion more than offsets the negative buoyancy due to water
loading. Therefore, both contributions, or neither contribution,
should be considered when assessing parcel buoyancy.
Correction factors for ice-phase transitions (e.g., Reuter and
Beaubien 1996; Rivas Soriano and García Díez 1997) and
sphericity terms (Shutts 1990, p. 2747) also may be included to
assess MSI, but these generally are not performed. Doswell and
Rasmussen (1994), in an approach distinct from Xu and Emanuel (1989),
present arguments for neglecting some of these effects in the
calculation of CAPE from observations, while retaining the correction
for virtual temperature. Since the use of CAPE/SCAPE from a
forecasting perspective does not require numerical precision, these
additional corrections are probably not necessary. If numerical
precision is required, however, all effects should be accounted for.
In this article, we have not included explicitly the
virtual-temperature correction for simplicity, but we recognize that,
for certain applications (e.g., warm-season events, deep tropospheric
circulations), it should be incorporated.
3f The absence of instability
Finally, if both adequate moisture and lift are present in the absence
of MSI, such that ascending air is forced to its condensation level
and beyond (forced convection), banded clouds may still form, with
heavy precipitation being the result [e.g., the case presented in
Doswell et al. (1998, section 3c)]. Forced slantwise ascent leading
to a single cloud/precipitation band can occur in the absence of MSI
over a mountain due to orographic lift or over a frontal zone due to
secondary circulations associated with frontogenesis, but this is not
free convection. When MPVg is positive, multiple bands can only be
generated externally by preexisting PVg or MPVg anomalies (e.g.,
Chan and Cho 1989; Cho and Chan 1991; Xu 1992). Observational
documentation of these features, however, has not occurred.
Therefore, the absence of MSI does not preclude the formation of
single or multiple banded clouds and precipitation, much as the
absence of potential or conditional instability to moist gravitational
convection does not preclude the same.
3g Assessing MSI from observations
Although numerous studies have used observations to assess MSI,
difficulties arise in doing so [see, for example, Persson (1995,
section 2)].
For instance, the assessment of MSI is sensitive to moisture, a
variable with strong spatial gradients and not measured with the
same resolution or accuracy as pressure, temperature, and wind.
Another difficulty is that the typical spacing of Mg surfaces is
less than the spacing between upper-air stations, such that the
horizontal variations in thermodynamic variables along Mg surfaces is
poorly resolved.
In order to deal with these limitations, previous researchers have
resorted to using a single observational sounding and assuming
geostrophy (e.g., Emanuel 1983b; Byrd 1989), estimating the vorticity
with height (e.g., Seltzer et al. 1985; Reuter and Aktary 1993), or
taking direct observations along M surfaces using aircraft (Emanuel
1988).
Also, like gravitational instability, the rapid time scale of
adjustment to slantwise neutrality is believed to occur very quickly
( ~ 3 h or less) meaning that standard atmospheric observations
will not capture the thermodynamic structure of the adjustment.
Therefore, conclusions about many of the details involved in slantwise
convection necessarily must be inferred.
Using gridded numerical datasets from objective analysis or mesoscale
models may appear to sidestep these issues, but the use of these
datasets presents its own problems (see section 7). The
analyst must be able to recognize the limitations of the particular
dataset chosen and act accordingly.
4 Frontogenesis and MSI
The eventual release of MSI is predicated upon slantwise parcel
lifting beyond the lifting condensation level to the level of free
slantwise convection (LFSC). Therefore, saturation must be present in
the region of slantwise ascent in order for the instability to be
released. Typically, previous authors examine the relative humidity
and if it is greater than some threshold (say, 80%), then saturation
is considered to have occurred or is imminent. This value is smaller
than 100% to account for data errors, inadequacies in the model (see
section 7), and/or saturation with respect to ice.
The ascent required to lift a parcel forcibly to its LFSC can
arise from frontogenetical circulations, traveling meso- or
synoptic-scale systems, orography, or any other mechanism of
sufficient magnitude. Doswell (1987, 7) has argued that
synoptic-scale ascent probably is not responsible for initiation of
deep, moist gravitational convection. On the other hand, slantwise
convection occurs on slightly larger scales than gravitational
convection. Therefore, resolving the processes responsible for lift
on the meso-a and synoptic scales (greater
than 200 km) is likely in most cases of suspected MSI. The relative
roles of synoptic and mesoscale processes in the initiation of
gravitational and slantwise convection is poorly known, compounding
the difficulty in understanding convective initiation (Ziegler and
Rasmussen 1998). The details of convective initiation, however, are
beyond the scope of this review.
In comparing observations to idealized models, it is useful to
draw the distinction between the release of MSI occurring due to the
growth of infinitesimal perturbations (i.e., normal-mode theory) and
the release of MSI occurring in the presence of some larger-scale
forcing (i.e., frontogenesis, orographical circulations, etc.).
Fischer and Lalaurette (1995a,b) compare these scenarios, finding
that idealized normal-mode simulations produce growth rates that are
too slow [ ~ (11 h)-1] to
account for observed features. Also, weaker vertical velocities
develop in the normal-mode simulations compared to simulations of MSI
with frontogenesis. Strong forcing (say, in the form of
frontogenesis) is required to overcome two factors that inhibit the
release of MSI: turbulent diffusion in the narrow, moist ascent and
the resistance of the positive buoyancy in the broader, dry,
compensating subsidence. Therefore, finite-amplitude forcing is
usually considered to be present for the release of MSI in the real
atmosphere [see also Innocentini and dos Santos Caetano Neto (1992)].
These studies support the observation that few examples of MSI in the
absence of frontogenesis exist [a possible exception is Wood and
Nielsen-Gammon (1994)].
Because both frontogenesis and slantwise convection due to the release
of MSI produce banded precipitation, some authors try to distinguish
between banded precipitation purely due to frontogenetical forcing
from those purely due to the release of MSI (e.g., Seltzer et
al. 1985; Snook 1992; Rauber et al. 1994). We argue in this section
that the atmospheric response in an environment characterized by MSI
is typically closely related to the frontogenetic forcing, making this
separation intractable (Thorpe and Emanuel 1985, 1821-1822;
Emanuel 1994, 412).
First, numerous published observational examples demonstrate the
simultaneous presence of weak symmetric stability and frontogenesis,
suggesting that slantwise neutrality has been reached. The most
convincing of these studies include Emanuel (1983b, 1988), Sanders and
Bosart (1985), Sanders (1986), Colman (1990a,b), Reuter and Yau
(1990), Thorpe and Clough (1991), Locatelli et al. (1994),
Kristjánsson and Thorsteinsson (1995), Huo et al. (1995), and
Loughe et al. (1995).
The idealized-modeling studies of Emanuel (1985), Thorpe and Emanuel
(1985), and Xu (1989b) clarify the relationship between symmetric
instability and frontogenesis, implicit in the Sawyer-Eliassen
equation for secondary frontal circulations. [Reviews of the
Sawyer-Eliassen equation can be found in Bluestein (1986), Eliassen
(1990), and Keyser (1998).] In the Sawyer-Eliassen equation, the
symmetric stability (through PVg) modulates the atmospheric
response to the forcing (i.e., the same forcing produces narrower,
stronger ascent in an environment of weaker symmetric stability than
in an environment of stronger symmetric stability). As such,
separating the contributions to ascent from the release of MSI versus
frontogenesis is not possible within the framework of the
Sawyer-Eliassen equation.
Second, as discussed in Emanuel (1994, sections 11.2 and 12.3) and
Mapes (1997), it is useful to consider two types of (gravitational or
slantwise) convection: activated (or triggered) and
statistical-equilibrium. Activated convection requires a build-up of
available potential energy before the convection is ``triggered'' by
some lifting mechanism, thereby releasing the instability.
Statistical-equilibrium convection, on the other hand, is a process by
which the consumption of available potential energy due to convection
occurs as physical processes, such as differential horizontal thermal
advection in the vertical, tend to destabilize the atmosphere. Thus,
statistical-equilibrium convection occurs in an environment in which
CAPE/SCAPE is relatively constant in time.
Whereas activated moist gravitational convection is commonly observed
in the atmosphere, clear observational signatures of activated
slantwise convection are not as readily identifiable. In activated
moist gravitational convection, the build-up of large CAPE is often
aided by the presence of a low-level capping inversion, which is
eventually penetrated by surface-based ascent leading to the release
of the instability. Therefore, by analogy, activated moist slantwise
convection would be indicated by a region of SCAPE prior to the onset
of slantwise convection. Few studies, however, thoroughly document
the conditions before slantwise-convective initiation.
In contrast, statistical-equilibrium slantwise convection appears to
be more commonly observed than activated slantwise convection (Emanuel
1994, 410-412), particularly along frontal zones, where increases in
SCAPE are nearly offset by the consumption of SCAPE by ascent along
the frontal zone. A notable example is Wolfsberg et al. (1986), in
which geostrophic differential lapse-rate advection maintains the MSI
over 12-24 h, even in the presence of continued slantwise ascent. In
fact, slantwise convection may appear to persist for many hours after
moist symmetric neutrality is reached, suggesting that frontogenesis
may continue to force ascent (e.g., Sanders and Bosart 1985; Saitoh
and Tanaka 1987; Lemaître and Testud 1988).4 Many cases
of near-slantwise neutrality appear to support the existence of
presumed slantwise convection in the presence of frontogenesis
(previously listed); large negative values of MPVg are not observed
and the Mg and qe surfaces are nearly parallel, suggesting
that equilibration is likely to be rather efficient. Indeed, Reuter
and Yau (1990) claim that the observed adjustment time is three hours
or less, consistent with the rapid equilibration time (f-1) from
scaling arguments (Emanuel 1983b, p. 2032). It should be reiterated
that statistical-equilibrium slantwise convection does not need to
imply that SCAPE is zero necessarily, only that it is relatively
constant. Consequently, measures of PSI may be more appropriate than
measures of CSI in these situations.
Finally, for moist gravitational convection, the nature of the
large-scale forcing and the response (resulting circulation), and the
time scales over which they operate, are so different (hours versus
minutes, respectively) that it is relatively easy to separate forcing
from response. In contrast, for slantwise convection, time scales for
frontogenesis and the release of MSI are more similar (hours), making
this scale separation more difficult.
5 The coexistence of moist gravitational and moist symmetric
instabilities
It is often observed in the atmosphere that regions of moist
gravitational instability (CI or PI) may be in the same vicinity as
regions of MSI (CSI or PSI) (e.g., Emanuel 1983b, Fig. 6; Bennetts and
Ryder 1984, Fig. 8; Donaldson and Stewart 1989, Figs. 8 and 13; Thorpe
and Clough 1991, Fig. 2; Lemaître and Scialom 1992, Fig. 12;
Martin et al. 1992, Fig. 10; Snook 1992, Fig. 10; Reuter and Yau 1993,
Figs. 6, 9, 11, and 13; Lagouvardos et al. 1993, Figs. 7-8; Martin
1998, Figs. 8a, 11a, and 12a).
For example, a typical stability profile across a frontal environment
is illustrated schematically in Fig. 4, based on an
unpublished design by J. Moore and S. Nolan. In the warm sector, CI
may exist, whereas closer to the front, CSI, symmetric neutrality, and
weak symmetric stability may be present (Fig. 4).
As discussed in section 3b, CI is a special case of CSI in
which qe* surfaces not only tilt more steeply than Mg
surfaces, but are overturned, such that
¶qe* /¶z < 0. Likewise, PI is a special case of PSI in which
qe surfaces not only tilt more steeply than Mg surfaces,
but are overturned, such that ¶qe / ¶z < 0.
As such, blindly employing the tests for CSI (MPVg* < 0 and the
Mg-qe* relationship) will identify regions of CI and
blindly applying the tests for PSI (MPVg < 0 and the
Mg-qe relationship) will identify regions of PI.
Whereas there have been some attempts to discriminate between
situations characterized by CI/PI from those characterized by CSI/PSI
(Bennetts and Sharp 1982, 598-599; Moore and Lambert 1993;
Wiesmueller and Zubrick 1998, 86), this distinction is not always made
(e.g., Gyakum 1987, p. 2339; Lemaître and Scialom 1992, Fig. 12;
Lagouvardos and Kotroni 1995, Fig. 10; Chen et al. 1998, Fig. 21) or
is made, but not applied properly (e.g., Moore and Blakley 1988,
p. 2170, Fig. 19; Wang et al. 1990, Figs. 1 and 8; Shields et
al. 1991, 956-959; Davidson et al. 1998, p. 1623).5
That MPVg can be used to identify PI and PSI led McCann (1995, 801)
to refer to MPVg as an ``all-purpose convection diagnostic tool''
to locate regions where the atmosphere is susceptible to moist
gravitational or symmetric instability, seemingly without regard to
the nature of any resulting convection. Without considering the
distinction between CI/PI, CSI/PSI, and inertial instability leads to
nonsensical statements such as, ``The conditions for CSI are satisfied
. . . via inertial and conditional instability, rather than
through inertial and conditional stability. . . .'' (Davidson et
al. 1998, p. 1623; italics are present in the original citation).
Furthermore, McCann's (1995, 801) statement that ``the effects of
equal CAPE and SCAPE are probably about the same, since parcel
accelerations are equal'' implies that vertical and horizontal
accelerations of similar magnitudes produce similar dynamics in the
atmosphere, a misinterpretation of the differing dynamics of moist
gravitational and symmetric instabilities (see section 3d).
In order to be precise, we strongly recommend assessing CI/PI and
inertial instability prior to assessing CSI/PSI, in any given case.
5a Convective-symmetric instability
Ultimately, a deeper understanding of how convection (gravitational,
slantwise, or both) organizes in the presence of both CI/PI and
CSI/PSI is sought. We should note that Xu and Clark (1985) argue for
a continuum between gravitational and slantwise convection, so, in a
sense, the distinction that is drawn between gravitational and
slantwise convection can be considered arbitrary. As further noted by
Jones and Thorpe (1992, 242), ``the strong distinction which is often
made between flows with positive and negative potential vorticity is
an artefact [sic.] of the use of balanced equations, rather than
a physical property of atmospheric flow.''
Nevertheless, we begin by noting that as an initially gravitationally
and symmetrically stable baroclinic atmosphere is destabilized by, for
example, surface heating or increasing the vertical shear of the
geostrophic wind, CSI/PSI will arise before CI/PI (Emanuel 1994,
410), but owing to the larger growth rate and energy release of moist
gravitational convection compared to slantwise convection,
gravitational convection, if initiated, is likely to dominate in time
(Bennetts and Sharp 1982, 598-599).
Following Emanuel (1980, 220, 245-250), Jascourt et al. (1988,
188-189) term the situation where CI/PI and CSI/PSI coexist
convective-symmetric instability. Therefore, the question arises as
to the mesoscale circulations in the atmosphere to organize any
resulting convection in such an environment.
Xu (1986a, 331) proposes two mechanisms for rainband development,
mechanisms we now recognize as forms of convective-symmetric
instability. The first he refers to as ``upscale development,'' where
small-scale moist gravitational convection develops first, followed by
mesoscale banded organization of clouds due to the release of
symmetric instability as the environment becomes gravitationally
stabilized. It seems that this type of development would be most
likely to occur outside of frontal regions where small-scale moist
gravitational convection organizes in the absence of synoptic-scale
airmass boundaries. In contrast, Xu (1986a) refers to ``downscale
development,'' where bands generated during frontal ascent in a moist
symmetrically unstable environment lead to latent-heat release,
effectively destabilizing the midtroposphere to gravitational
convection. Eventually, the release of moist gravitational
instability leads to band formation. Xu's (1986a) downscale
development is similar to the three-stage process of
frontal-precipitation-band development hypothesized by Bennetts and
Hoskins (1979, 961-962).
A likely observational example of upscale development in a situation
of convective-symmetric instability is documented by Jascourt et
al. (1988). From a region of scattered cumulus over northern
Louisiana, five parallel cloud bands simultaneously grew to become
lines of thunderstorms. The bands were aligned along the 700-500-mb
shear, a layer in which the moist symmetric stability was especially
weak. The vertical stratification in the lower troposphere, however,
was conditionally unstable to gravitational convection with CAPE of
more than 1000 J kg-1. Jascourt et al. (1988) hypothesize that
the initial latent-heat release by the scattered cumulus in the layer
of weak symmetric stability favored the development of
convective-symmetric instability and organized the convection into
the five bands. This case suggests that the nature and
organization of convection can be modulated by the symmetric
stability.
An alternate scenario for the release of upscale
convective-symmetric instability is modeled by Zhang and Cho (1992),
Seman (1994), and Bélair et al. (1995). Zhang and Cho
(1992) and Bélair et al. (1995) use mesoscale
numerical-model simulations of real squall lines to demonstrate how
the typical structure of a squall line [a schematic of which is
presented in Houze et al. (1989, Fig. 1)] acts to release
both PI and PSI simultaneously, illustrated schematically in
Fig. 5a, adapted from Seman (1991,
1992). The convective line reduces the moist gravitational
instability, whereas remnant negative MPVg is
transported back toward the trailing precipitation region, where the
release of PSI in the ascending front-to-rear flow helps to enhance
precipitation (Fig. 5a). Despite the
potentially large impact that the convective parameterization scheme
may have in these studies (e.g., Molinari and Dudek 1992), these
simulations appear to be consistent with the observation that the
convective line is often characterized by lapse rates that are moist
absolutely unstable (Fritsch and Bryan 1998), such that
MPVg* is less than zero. These results
are, in turn, consistent with those of Seman (1994), who shows that
convection in an idealized environment similar to that of Jascourt et
al. (1988) results in nearly upright updrafts. Slantwise ascent
then occurs, releasing symmetric instability, followed by downdrafts
that descend following sloping (dry and/or moist) isentropes
(Fig. 5a). Comparison to a barotropic
simulation (PI present only) indicates that the baroclinicity enhances
the mesoscale circulation leading to more intense, longer-lived
updrafts and more precipitation, consistent with the results of Xu
(1986a). Case studies of upscale development in the presence of
convective-symmetric instability and inertial instability are also
presented by Blanchard et al. (1998).
Whether the trailing precipitation region behind a mesoscale
convective system, oftentimes referred to as the stratiform region, is
characterized by stratiform or convective precipitation is being
debated.6 The results of Zhang and Cho (1992) and
Bélair et al. (1995), if general to mesoscale convective systems,
would indicate that this region is characterized by slantwise
convection due to the release of MSI. Indeed, further observational
and modeling studies continue to suggest regions of MSI in the
trailing precipitation region of squall lines (e.g., Jiang and
Raymond 1995; Braun and Houze 1996). Therefore, referring to this
region as ``stratiform'' (i.e., occurring in a stable environment) may
have to be reconsidered.
Observed inhomogeneities in vertical motion and precipitation rate
along frontal zones in the form of embedded bands or cells may suggest
manifestations of downscale convective-symmetric instability. As the
amount of moisture available to the circulation increases, the
likelihood of generating embedded moist gravitational convection
increases (Saitoh and Tanaka 1988). In a frontal environment
initially characterized by PSI, Bennetts et al. (1988, 368-369) and
Locatelli et al. (1994, Fig. 13) argue that the circulation associated
with an embedded band will overturn contours of qe, thereby
leading to PI, a result replicated in idealized models by Saitoh and
Tanaka (1987), Innocentini and dos Santos Caetano Neto (1992), and
Persson and Warner (1995). On the other hand, observational (Thorpe
and Clough 1991) and modeling (Holt and Thorpe 1991; Dudhia 1993;
Fischer and Lalaurette 1995a; Persson and Warner 1995) research shows
that Mg surfaces can buckle, as well. But, as noted by Fischer and
Lalaurette (1995b, p. 1318), experimental distinction between these
two processes is difficult and indistinguishable in their final
states, so the difference is probably academic.
A possible example of downscale convective-symmetric instability is
Neiman et al.'s (1993) elevator/escalator concept for warm-frontal
ascent (Fig. 5b) in which isolated regions of strong
sloping ascent (45° to the horizontal) 10 km wide (the
``elevator'') contrast with weaker regions of gentler slantwise ascent
(10° to the horizontal) roughly 15 km wide (the ``escalator'').
Reuter and Yau (1993, 378) determine that the warm-frontal environment
that Neiman et al. (1993) analyze is characterized by both PI and PSI,
suggesting that the release of PI may be occurring in the ``elevator''
convective elements, while the release of PSI may be occurring in the
``escalator'' slantwise regions. Parsons and Hobbs (1983, p. 2385),
Bennetts and Ryder (1984, Fig. 16), Byrd (1989, p. 1127), and
Colman (1990a,b) also observe similar convective structures embedded
in slantwise ascent in an environment characterized by both PI and
PSI. Rainband studies before the advent of MSI theory in the early
1980s (e.g., Browning et al. 1973) and the idealized modeling work of
Xu (1989b, Figs. 16 and 18) also may indicate such a process. These
precipitation structures may represent a form of downscale
convective-symmetric instability, where both moist gravitational and
moist slantwise convection occur in an environment characterized by
both moist gravitational and moist symmetric instabilities. That
these precipitation structures commonly are observed may suggest that
convective-symmetric instability is not atypical, as demonstrated,
for example, by the large number of soundings from central Alberta
exhibiting both moist gravitational and moist symmetric instabilities
(Reuter and Aktary 1995, Table 7).
5b Deep convection, hurricanes, and lightning
It is sometimes stated that the existence of deep cumulonimbus, large
precipitation rates, strong downdrafts, or lightning (i.e.,
thunderstorms) precludes moist slantwise convection (e.g., Bennetts
and Sharp 1982, 598-599). We argue below that insufficient evidence
exists to make such claims.
As in moist gravitational convection, moist slantwise convection
can result in vertically shallow or deep circulations, depending on
the thickness of the unstable layer and the level of neutral buoyancy
(also known as the equilibrium level). Therefore, it is possible for
slantwise convection to develop deep circulations. For example, the
outward slope of the upper eyewall of hurricanes (e.g., Marks et
al. 1992, Fig. 12f; Black and Hallett 1998, Fig. 10)
suggests the presence of slantwise convection (e.g., Emanuel 1989;
Black et al. 1994; Mathur 1997).
As discussed previously (for example, in reference to
Fig. 5a), updrafts of slantwise convection will ascend
nearly along the saturated moist adiabat, whereas downdrafts descend
dry adiabatically, overcoming the resistance of the positive buoyancy
and compensating for the ascent (e.g., Marécal and Lemaître
1995, 301). Therefore, processes that cool the descending air would
accelerate development of the slantwise circulation. Since
sublimation of snow is more efficient, in general, than evaporation of
rain in lowering the air temperature (Clough and Franks 1991), strong
mesoscale downdrafts are most likely created by sublimating snow. In
some observational and modeling research, sublimation of snow into dry
downdrafts, possibly also influenced by precipitation loading, has led
to saturation and vigorous descent (e.g., Ramstrom 1990; Stensrud et
al. 1991; Thorpe and Clough 1991, 929-930; Emanuel 1992; Marécal
and Lemaître 1995; Parker and Thorpe 1995; Zhang and Cho 1995;
Braun and Houze 1997), approaching 20 m s-1-1 in some situations (Black
et al. 1994). Thus quite strong circulations can result from
slantwise ascent/descent due to the inclusion of diabatic effects,
although the relative frequency of these more impressive vertical
motions remains relatively unknown.
In isolated thunderstorms, it is generally accepted that strong
updrafts exceeding 5 m s-1-1 favor coexisting ice and water phases that
appear to be required for the generation of lightning. This would
appear to exclude slantwise convection as being associated with
lightning, since most slantwise updrafts are assumed to be weaker
(tens of cm s-1-1 to a few m s-1-1), although direct observations are
lacking.
On the other hand, Williams (1991, p. 2512) notes that the mechanisms
that lead to charge separation are, in principle, independent of the
existence of moist gravitational instability, indicating the
possibility that lightning could be associated with slantwise
convection. We currently recognize three environments characterized by
MSI in which lightning is observed to occur.
The first environment characterized by both MSI and lightning is the
trailing precipitation region of mesoscale convective systems. As
discussed in section 5a, numerical and observational evidence
indicates the presence of MSI in the trailing precipitation region,
consistent with the occurrence of slantwise convection. The evidence
for lightning in this region is reviewed by MacGorman and Rust (1998,
275-277).
The second environment is that of wintertime convection. While some
cases of wintertime convection apparently are associated with CI
(e.g., Engholm et al. 1990) or with convective-symmetric instability
(e.g., Holle and Watson 1996), Colman (1990b, 1991) and Freedman
(1995) argue for the possible coexistence of slantwise convection and
lightning. MacGorman and Rust (1998, 292) summarize their review of
lightning in winter storms with, ``we are aware of no thorough
scientific investigation of causal relationships between the
electrical state of winter storms and their snowfall. Extensive tests
to evaluate the proposed hypotheses concerning possible links between
lightning and the mesoscale and synoptic scale meteorology associated
with winter storms have yet to be performed.''
Finally, lightning has been observed in the eyewall during the
deepening phase of mature hurricanes, although rarely (e.g., Molinari
et al. 1994, 1999). As discussed previously, the eyewall can be
treated as symmetrically neutral and is generally free of lightning
activity. Therefore, lightning in the eyewall may indicate an
outbreak of buoyancy-driven updrafts and temporary disruption of
symmetric neutrality, resulting in intensification of the hurricane
(Molinari et al. 1994, 1999).
Perhaps noncoincidently, these three environments also tend to be
characterized by the occurrence of higher-than-normal percentages of
positive cloud-to-ground lightning. Although not the only mechanism
posited for the occurrence of positive lightning, some believe that
strong vertical wind shears horizontally separate the cores of
differently signed charges, thereby exposing positive charge aloft to
the ground (e.g., Orville et al. 1988; MacGorman and Rust 1998, 275,
290). We suggest that these environments also may be associated with
MSI. This hypothesis, however, has not been rigorously defended.
Williams (1991) notes that evaluating MSI in these cases can be
difficult, particularly eliminating CI completely from consideration.
Nevertheless, coupled with our incomplete understanding of the
conditions under which cloud electrification occurs (Vonnegut 1994),
we do not know enough at this time to state conclusively that the
existence of deep cumulonimbus, large precipitation rates, or
lightning precludes the existence of slantwise convection. In fact,
some evidence suggests otherwise.
6 Nature of the banding
Perhaps the most operationally significant use of MSI is to assess the
nature of the precipitation banding (orientation, spacing, number of
bands, movement, etc.) that may occur. The goal in operational
forecasting is to anticipate the occurrence and nature of the large
gradients in precipitation that can result if these bands remain
nearly stationary.
MSI theory makes certain predictions about the size, shape, movement,
orientation, wavelength, and the existence of single or multiple
bands. Yet, few studies evaluate those parameters as a test of
whether MSI may have been associated with their cases of banded clouds
and precipitation.
In that regard, characteristics of banded clouds and precipitation in
order to be considered associated with MSI are enumerated below
[adapted from Seltzer et al. (1985, p. 2208)].
- The bands and their environment should be nearly two-dimensional
(i.e., be nearly ``symmetric''). The more two-dimensional the bands
and their environment are, the better symmetric-instability theory
will apply.
- The condition for MSI should be met in the region of the bands.
- The bands should be aligned nearly along the thermal wind and
should be strongest in the region of the instability. Bands can
deviate from the direction of the thermal wind, however, by as much as
15° anticyclonically in idealized models (Busse and Chen 1981;
Miller and Antar 1986; Jones and Thorpe 1992; Zhang and Cho 1995; Gu
et al. 1998) or greater in observations (e.g., Byrd 1989, Fig. 8;
Colman 1990b, Fig. 17).
- The bands, if they are moving, should be moving with the
environmental flow (i.e., bands are advected by the flow; they do
not propagate relative to the environmental flow).
- The spacing between the bands should be related to the depth of the
unstable layer and the slope of the isentropes, as expressed in
Emanuel (1979) and Seltzer et al. [1985, (3.3)].
- The slope of the ascent in the region of the bands should lie
between the slopes of moist adiabatic and Mg surfaces. When the
flow is hydrostatic, the ascent will occur exactly on the isentropic
surfaces.
In this section, we review previous work attributing banding of clouds
and precipitation to MSI and evaluate their methodologies in search of
useful methods to predict banding of clouds and precipitation.
Previous observational studies of banded precipitation are summarized
also by Lagouvardos et al. (1993, Table 1).
Seltzer et al. (1985) examine 15 banded and nonbanded precipitation
events (as determined by Doppler radar) over New England and find
substantial agreement between theory and their observations,
indicating that MSI is likely associated with the banded precipitation
in these events. The degree of banding appears to be strongly related
to the magnitude of the maximum wind shear with better-defined banding
more likely for shears exceeding 13 m s-1 km-1. They also
show that the band orientations are close to the 1000-500-mb
thickness contours and the observed wavelengths agree well with the
predicted values from theory for most cases. Problems with large
ageostrophic shear and curvature, and assessing geostrophy from the
sparse soundings in some of their cases, may have limited the success
of their study.
Another limitation of Seltzer et al. (1985) is that the lifting
mechanism is not identified, as they explicitly attempt to eliminate
the effects of frontogenesis by requiring surface fronts and low
pressure centers to be more than 500 km away from the region of
analysis. Sanders (1986) notes a potential pitfall in this approach,
however. Reanalyzing one of Seltzer et al.'s (1985) cases, Sanders
(1986) finds that lower-tropospheric frontogenesis is likely occurring
in the region of banded precipitation northwest of the surface low
center, a condition that Seltzer et al. (1985) explicitly try to
eliminate from their cases. Unfortunately, published studies of
similar synoptic situations exist in which the authors do not
calculate frontogenesis (e.g., Snook 1992; Reuter and Nguyen 1993).
Consequently, these studies may be susceptible to the same pitfall,
reiterating the importance of calculating forcing for ascent for
individual cases in the vicinity of where MSI might be present.
Therefore, since forcing for ascent need not be surface based, but can
exist above the boundary layer, the absence of analyzed surface fronts
is not adequate for eliminating the possibility of frontogenetical
forcing for ascent.
Further examples of climatologies of banded clouds and precipitation
include Bennetts and Hoskins (1979), Bennetts and Sharp (1982), and
Byrd (1989).
Bennetts and Hoskins (1979, section 5) examine 36 cases of satellite
and radar data and do not find a strong relationship between
Richardson number and banding.
Bennetts and Sharp (1982, section 4) consider 30 cases of banded
precipitation and 14 cases of nonbanded precipitation. They note
that, for large values of the MSI growth-rate parameter, the
precipitation is likely to be banded, whereas for small positive
values and negative values, the growth-rate parameter is not very
useful in discriminating banded from nonbanded precipitation.
Byrd (1989) finds similar results in 27 ``overrunning'' precipitation
events (226 soundings) over the southern Plains. He notes that over
80% of his banded and strongly-banded cases meet the
Richardson-number criteria for MSI, but nearly 60% of the nonbanded
cases do also, suggesting limited utility of this parameter for
discrimination, in agreement with Bennetts and Sharp (1982). A
complication is that his strongly-banded category has higher
incidences of PI (Byrd 1989, p. 1127), perhaps suggesting the
occurrence of banded convection associated with the release of pure
moist gravitational instability or convective-symmetric instability
(section 5a). Byrd (1989, p. 1124) also noted stronger
wind shear in the boundary layer for strongly-banded events.
This body of research suggests that well-constructed climatologies of
even a small number of events [as suggested by DeVoir (1998)] may
produce useful results, evaluating the likely existence of slantwise
convection associated with MSI.
Whether single or multiple bands form in the presence of frontogenesis
is addressed using idealized numerical models.
As discussed in section 4, the results of Emanuel (1985) and
Thorpe and Emanuel (1985) indicate that weak positive symmetric
stability in the warm air in the presence of frontogenesis leads to a
single band of ascent that narrows as the symmetric stability
approaches neutrality. In general, the inviscid Sawyer-Eliassen
equation cannot be solved when PVg (or MPVg, when moist) is
negative. Adding viscous effects to the Sawyer-Eliassen equation (Xu
1989a), however, permits solutions when PVg (or MPVg, when
moist) is negative. Therefore, using a viscous form of the
Sawyer-Eliassen equation in the presence of localized frontogenetical
forcing, Xu (1989b) finds a single band of ascent for positive
symmetric stability.
As the MPVg is decreased toward zero and becomes negative, the
single band becomes narrow and intense (Figs. 6a,b).
If the forcing becomes horizontally widespread and MPVg is
negative, multiple bands become embedded within the larger-scale
frontal circulation, consistent with Knight and Hobbs (1988, 926).
If the forcing remains localized and MPVg is decreased further, the
multiple bands become more intense and more widely spaced
(Figs. 6c,d).
These idealized modeling experiments help to explain the observations
of Bennetts and Sharp (1982) and Byrd (1989) that many nonbanded
situations may exceed the criteria for MSI.
These experiments further suggest that a balance between the magnitude
and areal extent of the frontogenetical forcing, the eddy viscosity,
the moisture availability, and the value of the MPVg exists that
determines whether single or multiple bands form, but observational
verification of these idealized-modeling experiments has not been
forthcoming.
Based on the results of this observational and modeling work, it is
clear that, in a forecasting mode, even if a region of the atmosphere
meets the criteria for MSI, the formation of banded structures cannot
be assumed a priori. On the other hand, the existence of banded
structures, and the validation of the observed structures to those
predicted by MSI theory, is strong evidence for the bands being
associated with the release of MSI. The above results also indicate
that, even in the presence of frontogenesis, multiple bands can form,
although the conditions under which single versus multiple bands would
form have not been validated in a rigorous way at this time.
Therefore statements such as ``the [existence of] multiple bands
. . . indicate[s] that CSI is a more likely candidate for the observed
precipitation than frontogenetical forcing'' (Snook 1992, 438) are not
accurate.
7 MSI in mesoscale-model simulations
The growth in availability of quality gridded atmospheric datasets
(e.g., Keyser and Uccellini 1987) makes determination of MSI in those
datasets relatively easy (e.g., Bennetts and Sharp 1982; Snook
1992; Grumm and Nicosia 1997; Martin 1998; Wiesmueller and Zubrick
1998). A reasonable question is whether MSI and its attendant
circulations can be assessed accurately in these datasets. Crucial
tests of the effect of horizontal and vertical resolution on unforced
(no frontogenesis, radiative, or surface forcing acting on the MSI,
i.e., no externally applied lifting mechanism), hydrostatic, nonlinear
MSI in a mesoscale model are performed by Persson and Warner (1991,
1993) and Ducrocq (1993). They find that horizontal/vertical grid
spacings greater than 30 km/0.34 km are inadequate to
resolve the resulting circulations explicitly. To capture the most
unstable mode, grid spacings no greater than 15 km/0.17 km
are required. If the most unstable mode is not adequately resolved,
then the resulting evolution of the MSI circulations is substantially
slower and the amplitude reduced from what would be expected in the
real atmosphere. Even in the presence of lower-tropospheric
frontogenesis, these results are consistent with those of Knight and
Hobbs (1988), who find that hydrostatic nonlinear MSI bands are absent
at 80-km horizontal grid spacing, poorly resolved at 40 km, and
well-resolved at grid spacings 5-10 km.
In comparison, observations of banded structures indicate typical
widths of the bands of 20-100 km (e.g., Byrd 1989) and typical
distances between bands of 10-220 km, (e.g., Seltzer et al. 1985;
Byrd 1989; Lagouvardos et al. 1993). Therefore, consider a mesoscale
model with horizontal resolution of 20-90 km, values typical of the
short-range operational forecast models at the National Centers for
Environmental Prediction, simulating convection over a region with
MSI. In this scenario, the model may be able to resolve the
larger-scale frontogenetical circulation, if present, but not the
smaller-scale bands.
Inadequate model resolution prohibits explicit resolution of the
scales responsible for banded precipitation. Besides the
underprediction of maximum vertical velocities, a less vigorous
circulation in the model would decrease the region of saturation,
decreasing the likelihood of releasing heretofore untapped regions of
MSI. Therefore, modeled precipitation rates would be substantially
less than those observed, despite perhaps a nearly accurate simulation
of the precipitating region. An example of this situation is
presented in Martin (1998, 333, Fig. 6), who observes two 35-km
wide snowbands on radar, separated by 35 km and parallel to the
thermal wind, suggestive of the presence of MSI. Martin (1998)
examines the potential for the release of MSI in a mesoscale-model
simulation of this event and finds that the necessary conditions for
instability and lift are present, but inadequate moisture exists to
reach saturation. Hence, he concludes that, based on the model
simulation, MSI could not have been responsible for the observed
snowbands. His analysis, however, employs a mesoscale model with
40-km horizontal grid spacing. Although the model is too coarse to
resolve the observed snowbands, Martin (1998, 335) states that he
desires an accurate simulation of the narrow region of snowfall,
rather than an accurate simulation of the individual snowbands
comprising the event. Consequently, the coarse resolution of the
model results in the areal extent of the precipitation being
reasonably well-forecast, but the magnitude of the precipitation
maxima are underforecast by as much as 50% (Martin 1998,
cf. Figs. 1 and 4). Although many explanations may be
proposed for the underprediction of the precipitation maxima, Knight
and Hobbs (1988), Ducrocq (1993), and Persson and Warner (1991,
1993) show that the model resolution has a large impact on the ability
to simulate MSI circulations properly, results consistent with those
of Martin (1998).
Molinari and Dudek (1992, section 5) discuss the implications for
slantwise convection in numerical weather prediction models. Whereas
grid-resolvable precipitation is calculated by the large-scale
explicit precipitation schemes, convective precipitation due to the
release of moist gravitational instability is calculated by cumulus
parameterization schemes. Precipitation generated by the release of
MSI during slantwise convection, however, is neither grid-resolvable
nor convectively parameterized at resolutions greater than about
10 km. The inclusion of this missing effect may substantially
improve the numerical prediction of quantitative precipitation, as
suggested by Emanuel (1983a, p. 2375) and Persson and Warner
(1993, p. 1832). Balasubramanian and Yau (1995) apply a
parameterization for slantwise convection for an idealized three-layer
model of cyclogenesis in which they illustrate the importance of the
release of MSI along the warm and back-bent warm fronts, which aids in
deepening the surface cyclone. Their results are in agreement with
those of Cao and Cho (1995) who found that negative MPV is generated
first in the warm sector near the north end of the cold frontal zone,
then develops along the bent-back front at the mature stage. For a
simulation of a central United States cyclone, Lindstrom and Nordeng
(1992) apply Nordeng's (1987, 1993) parameterization of slantwise
convection patterned after the Kuo scheme for moist gravitational
convection, which produces neutrality to slantwise convection along a
M surface. Their results (Fig. 7) show that the parameterization improves the
simulation of the quantity and areal extent of precipitation
associated with a midwest United States cyclone, indicating the
potential usefulness of this approach. Also, the addition of the
slantwise-convective parameterization helps the model generate
divergent circulations more quickly (improving the so-called
model-spinup problem). Although Molinari and Dudek (1992, section 5)
suggest that slantwise convection could occur on the grid scale in
models, they also caution that their conclusions are based on limited
research results, which do not include the contemporaneous research of
Lindstrom and Nordeng (1992). Consequently, future research in this
area might result in improvements in quantitative precipitation
forecasting. Whereas operational implementation of slantwise-convective
parameterization in research-mesoscale and operational-forecast models
is less imperative with the push to smaller horizontal grid
resolutions, the climate-modeling community will have need to deal
with slantwise convection for the foreseeable future.
An additional concern for assessing MSI using mesoscale-model output
involves how the model is initialized. Observations of water
substance (vapor, cloud water, hydrometeors) in the atmosphere tend to
be more sparse than observations of the thermal, wind, or mass fields.
Therefore, models rely heavily on developing their own water fields.
If the model simulation is started as a static initialization (i.e.,
lacks cloud-water and precipitation fields at the initial time; the
so-called cold start), like many real-time (Mass and Kuo 1998,
Table 1) and research mesoscale models, then it will take time for the
model to spin up and generate those fields. Depending on the synoptic
situation and model resolution, estimates of the typical time for
model spinup to occur range from 1-12 hours. The model-spinup
dilemma may pose a problem for the interpretation of Martin's (1998)
results, as the time at which the assessment of MSI occurs (0600 UTC
19 January 1995) is only 6 h after model initialization time,
comparable to the model-spinup time. This is possibly an inadequate
amount of time to have fully-developed cloud and precipitation fields.
Therefore, it is likely that the relative-humidity fields may
underestimate the saturated area, which in turn would underestimate
the area susceptible to slantwise convection. This may be another
factor that contributes to the underestimate of the modeled
precipitation (Martin 1998, cf. Figs. 1 and 4). On the other hand,
the National Centers for Environmental Prediction operational forecast
models are run in a continuous assimilation cycle, which limits this
spinup problem.
A final point is that different numerical models reach saturation and
generate clouds at different relative humidities [an approach
described by Mathur (1983) and Mocko and Cotton (1995)]. For example,
in the version of the Eta model (as of November 1998), clouds begin to
develop at relative humidities of 95% over the ocean surface, ramping
down to 80% over the lowest 10 model levels, and 75% over land (G. DiMego, Q. Zhao, and E. Rogers 1998, personal communication; Zhao et
al. 1997, 699), 80% in the Nested Grid Model (NGM; Hoke et al. 1989,
331), but 100% in the Rapid Update Cycle (RUC; S. Benjamin 1998,
personal communication). It is also of import that grid-point values
of relative humidity in the Eta model rarely exceed 95% (G. DiMego
and E. Rogers 1998, personal communication). Knowing these critical
values is essential to assessing whether slantwise convection may be
present within mesoscale-model data. Proper use of model data for
assessing slantwise convection, especially early in the simulation
when model spinup may be important, mandates knowledge of the model
characteristics. If model output is used wisely, then we believe it
can be useful.
Thus, operational implementation of MSI techniques in the forecast
office tends to consist of the following methodology (Grumm and
Nicosia 1997, 21-22; Wiesmueller and Zubrick 1998,
section 7). First, an evaluation of the model output for areas
meeting the MSI criteria over a large area for up to two days in
advance is performed. If a particular area is susceptible to MSI,
then that area is monitored into the short-range period (0-12 h).
Since the model resolution is too coarse to resolve individual
precipitation bands, the forecaster then must rely more heavily on the
observations at this stage. Bands, if they form, can be monitored for
locally heavy precipitation amounts and short-term forecasts can be
issued. At this time, there does not appear a way to be more specific
about the location of bands before they form, akin to the difficulty
in forecasting individual thunderstorms prior to initiation.
8 Concluding summary
In conclusion, we first summarize our principal conclusions and
recommendations in section 8a, followed by directions for
future research in section 8b. This article concludes with
thoughts about the use of CSI (symbols and acronyms are defined in the
appendix) in research and operational forecasting practice in
section 8c.
8a Principal recommendations
The following points represent our basic tenets regarding MSI
discussed in this article.
- Like deep, moist gravitational convection, moist slantwise
convection requires the simultaneous presence of instability,
moisture, and lift. The absence of any one of these three is
sufficient to prevent moist slantwise convection from occurring
(section 2).
- The terms CSI and slantwise convection do not have
interchangeable meanings (section 2).
- Strictly, hydrostatically and geostrophically balanced
basic states for [`(qe*)] and Mg, respectively, are
required for determination of CSI. In practice, the restriction on
[`(qe*)] can be relaxed to qe*, but Mg should
not be relaxed to M (sections 3a and 3b).
- Analogies to CI and PI indicate that the definitions commonly
employed for CSI are really for PSI. Ideally, when assessing CSI,
qe* should be used; when assessing PSI, qe should be
used (section 3b).
- Owing to the potential confounds with assessing the
Mg-qe* relationship, we recommend that MPVg* or
another measure of CSI not dependent on cross-section orientation
(e.g., SCAPE), along with a measure of CI and inertial instability, be
employed to assess CSI (sections 3c and 5).
- Even if the atmosphere is determined to be stable to moist
slantwise and moist gravitational convection, banded clouds and
precipitation may still form owing to forced ascent
(section 3f).
- By definition, an environment characterized by CSI possesses a
horizontal gradient in qe* and vertical wind shear, likely
indicating a frontal zone. In an environment in which CSI and
frontogenesis coexist, it is improper to attempt to separate the
circulations due to CSI from the frontal circulation
(section 4).
- Blindly applying tests for CSI/PSI may result in the
identification of regions of CI/PI or inertial instability
(section 5).
- The coexistence of CSI/PSI and CI/PI, as well as adequate
moisture and lift, may result in a mixture of moist gravitational and
moist slantwise convection associated with the release of
convective-symmetric instability (section 5a).
- The existence of deep cumulonimbus, large precipitation rates,
strong downdrafts, or lightning is not an adequate discriminator
between the existence of moist gravitational and moist slantwise
convection (section 5b).
- The nature of banding in clouds or precipitation appears to be
only weakly related to measures of CSI. More attention should be
paid, however, to evaluating other parameters (band spacing, movement,
orientation, etc.) associated with bands suspected of being related to
CSI (section 6).
- The resolution of most research mesoscale and operational
forecast models is inadequate to resolve individual CSI circulations.
Consequently, care should be taken in assessing model data for CSI
(section 7).
8b Future research
The preceding sections have presented an overview of the current
knowledge and perspectives on CSI and slantwise convection.
Observational, idealized-model, and mesoscale-model studies have
contributed to our present understanding. Despite the progress, a
number of unresolved problems and issues can be identified and serve
as subjects for further consideration. Among the topics that require
further research are (1) assessing MSI, (2) nature of banding, (3)
slantwise-convective parameterization, and (4) convective-symmetric
instability and the general nature of atmospheric convection.
As illustrated in this review (particularly, section 3b),
the specific diagnostics used to assess MSI are often misused. In
particular, the choice of a particular thermodynamic quantity
(qe or qe* as measures of PSI or CSI, respectively)
can affect the assessment of instability. The relative advantages and
disadvantages of diagnosing PSI versus CSI have not been explored.
Although we have suggested using CSI rather than PSI, case studies
illustrating the structure and evolution of these fields prior to
convective initiation have not been examined in general. For example,
it is observed that moist gravitational convection can be manifest in
a variety of ways that include supercell thunderstorms, mesoscale
convective complexes, squall lines, and pulse (``airmass'')
thunderstorms. Perhaps this sort of variety with slantwise convection
is waiting to be explored. If that were the case, then it may be that
certain diagnostics would work better for given situations in
assessing various manifestations of slantwise convection. As an
example, we have suggested in section 4 that SCAPE may
be more useful to forecasting activated slantwise convection than
statistical-equilibrium slantwise convection, but this hypothesis has
not been tested operationally. Consequently, the relative merits of
these diagnostics remain untested and await further
research.7
Section 6 discussed the nature of banding from both an
observational and idealized-modeling perspective. Unfortunately,
studies that bridge the gaps between these two perspectives have not
been performed. For example, a variety of idealized-modeling
experiments of both forced and unforced MSI (e.g., Xu 1986a, 1989b,
1992; Zhang and Cho 1995; Arbogast and Joly 1998) suggest that a
useful relationship might exist for forecasting the nature of the
banding as a function of frontogenetical forcing, friction, moisture
availability, and stability, yet there have been no rigorous
observational or idealized-modeling attempts to validate this
hypothesis. Also, as noted by Jones and Thorpe (1992, 257),
idealized-modeling studies incorporating three-dimensional basic
states have not been adequately explored. Also, Zhang and Cho (1995,
59-60) discuss the importance of three-dimensional simulations for
obtaining more realistic banded precipitation. Toward this goal, Gu
et al. (1998) have found that two-dimensional nonlinear symmetric
circulations can be unstable in three dimensions and they resemble
some observed three-dimensional substructures embedded in frontal
rainbands.
As discussed in section 7, the addition of
slantwise-convective parameterizations to mesoscale numerical models
dramatically improved the forecast of the intensity and areal extent
of precipitation, in at least one case (Lindstrom and Nordeng 1992).
Consequently, slantwise-convective parameterizations should be
developed, tested, and, if found to be useful, implemented in
research-mesoscale, operational-forecast, and general-circulation
models with the aim of improving the prediction of quantitative
precipitation (section 7).
The concept of convective-symmetric instability
(section 5a) has not been examined in detail in the
previous literature, and, as a result, there is much to be learned
about convective-symmetric instability from both observational and
idealized modeling perspectives. Given the typical inhomogeneous
condition of the atmosphere, areas favorable for MSI may be juxtaposed
with areas favorable for gravitational convection (e.g.,
Fig. 4) such that convection can possess
characteristics of slantwise convection, gravitational convection, or
both, and may evolve from one form to the other. Consequently,
convection can manifest itself in a variety of ways, depending on the
environmental stability, lift mechanism, amount of moisture, and other
factors. Overlaying radar and lightning data with MSI diagnostics can
provide information about storm structure and evolution. The
deficiency in our knowledge of this area is reiterated by Mapes (1997,
355), who states that the role of mesoscale organization in
determining the amount (and nature) of the convection remains unclear.
In addition, Jones and Thorpe (1992, 251-252, 257) state that
three-dimensional effects appear to increase the susceptibility of
symmetric instability to PI, leading to small-scale structure along
the length of the bands. The issue of whether ``horizontal
convection'' caused by the release of inertial instability plays a
role also has been inadequately addressed. The work of Seman (1994)
and Blanchard et al. (1998) suggests several ways that the atmosphere
responds to situations where two or more instabilities are present.
These issues may force rethinking the nature of convection beyond
compartmentalizing atmospheric motions into either gravitational,
slantwise, or horizontal convections (e.g. Xu and Clark 1985).
8c Conclusion
Within this article, previous literature on MSI and slantwise
convection is reviewed. Drawing parallels to the familiar
conceptualizations of gravitational convection allows a
reconsideration of the extent to which slantwise convection is
understood and used in research and forecasting environments. Indeed,
the argument could be made that the near-omnipresence of baroclinicity
in midlatitudes, even during the warm season, requires an evaluation
of the potential for slantwise convection. An example where such
diagnosis might be useful is during the early and late stages of deep,
moist convection, when gravitational instability is likely to be
small. The dichotomy between gravitational and slantwise convection
is also apparent in numerical modeling where parameterizations for
slantwise convection presently are not included in the majority of
research-mesoscale and operational-forecast models. Consideration of
convective-symmetric instability appears to be a prudent step toward
a more general consideration of atmospheric convection. It is
tempting to speculate that combining the intellectual resources of
those studying meso- and synoptic-scale processes with those studying
convective-scale phenomena, from both the research and operational
communities, has the potential to lead to further improvements in our
understanding of convective phenomena.
Acknowledgments.
We have benefited considerably from the advice and comments of Michael
Baldwin, Steven Corfidi, Greg DeVoir, Paul Janish, Ann McCarthy, and
Drs. Daniel Keyser, Donald MacGorman, John Molinari, James Moore,
Richard Rotunno, David Rust, Terry Schuur, Charles Seman, Alan Thorpe,
and Jeff Trapp. Insightful reviews of earlier versions of this
manuscript were provided by George Bryan, Ronald Holle, and Drs. David Blanchard, Kerry Emanuel, Jack Kain, Robert Maddox, Jonathan
Martin, John Nielsen-Gammon, Paul Roebber, David Stensrud, Robert
Weisman, Earle Williams, and Qin Xu. Katherine Day (NOAA Library,
Boulder, Colorado) obtained lists of references at our request,
assuring a thorough bibliography to draw upon for this review. The
first author thanks Dr. Charles Doswell III for his invaluable
discussions/arguments on atmospheric convection and encouragement
during the preparation of this manuscript. Most of this research was
conducted while the first author was a National Research Council
Postdoctoral Research Associate at the National Severe Storms
Laboratory. This work is inspired by and dedicated to the late Dr. Bernard Vonnegut, who challenged us to scrutinize paradigms.
APPENDIX
List of Symbols and Acronyms
|
|
| CAPE | convective available potential energy
|
| CI | conditional instability
|
| cp | specific heat of dry air at constant pressure
|
| CSI | conditional symmetric instability
|
| f | Coriolis parameter
|
| g | gravity; subscript denoting geostrophic
|
| gr | subscript denoting gradient
|
| L | latent heat of vaporization
|
| LFSC | level of free slantwise convection
|
| M | absolute momentum defined as v+fx
|
| Mg | geostrophic absolute momentum defined as vg+fx
|
| MPV | moist potential vorticity defined as
g h·Ñqe
|
| MPVg | moist geostrophic potential vorticity defined as
g hg·Ñqe
|
| MPVg* | saturated geostrophic potential vorticity defined as
g hg ·Ñqe*
|
| MSI | moist symmetric instability
|
| NGM | Nested Grid Model
|
| PI | potential instability (also called convective instability)
|
| PSI | potential symmetric instability
|
| PV | potential vorticity defined as
g h·Ñq
|
| PVg | geostrophic potential vorticity defined as
g hg·Ñq
|
| qvs | saturation mixing ratio
|
| RUC | Rapid Update Cycle
|
| r | distance along radius of curvature
|
| SCAPE | slantwise convective available potential energy
|
| T | temperature
|
| v | component of wind in along-front direction
|
| vg | component of geostrophic wind in along-front direction
|
| vgr | component of gradient wind in along-front direction
|
| x | cross-front coordinate
|
| z | height coordinate
|
| Gd | dry adiabatic lapse rate
|
| Gm | moist adiabatic lapse rate
|
| zg | vertical component of geostrophic relative vorticity
vector
|
| h | three-dimensional absolute vorticity vector
|
| hg | three-dimensional geostrophic absolute vorticity vector
|
| q | potential temperature
|
| qe | equivalent potential temperature
|
| qe*, qes | saturated equivalent potential temperature
|
| qw | wet-bulb potential temperature
|
| Ñ | gradient operator in x, y, and z coordinates
|
| [`( )] | basic state |
REFERENCES
Arbogast, P., and A. Joly, 1998: Potential vorticity inversion of a
two-dimensional steady flow: Application to symmetric instability.
Quart. J. Roy. Meteor. Soc., 124, 317-339.
Balasubramanian, G., and M. K. Yau, 1995: Explosive marine
cyclogenesis in a three-layer model with a representation of slantwise
convection: A sensitivity study. J. Atmos. Sci., 52, 533-550.
Barnes, S. L., F. Caracena, and A. Marroquin, 1996: Extracting
synoptic-scale diagnostic information from mesoscale models: The Eta
model, gravity waves, and quasigeostrophic diagnostics. Bull. Amer. Meteor. Soc.,
77, 519-528.
Bélair, S., D.-L. Zhang, and J. Mailhot, 1995: Numerical
prediction of an intense convective system associated with the July
1987 Montreal flood. Part II: A trailing stratiform rainband.
Atmos.-Ocean, 33, 475-500.
Bennetts, D. A., and B. J. Hoskins, 1979: Conditional symmetric
instability - a possible explanation for frontal rainbands. Quart. J. Roy. Meteor. Soc.,
105, 945-962.
Bennetts, D. A., and J. C. Sharp, 1982: The relevance of conditional
symmetric instability to the prediction of mesoscale frontal
rainbands. Quart. J. Roy. Meteor. Soc., 108, 595-602.
Bennetts, D. A., and P. Ryder, 1984: A study of mesoscale convective
bands behind cold fronts. Part I: Mesoscale organization. Quart. J. Roy. Meteor. Soc.,
110, 121-145.
Bennetts, D. A., J. R. Grant, and E. McCallum, 1988: An introductory
review of fronts. Part I: Theory and observations.
Meteor. Mag., 117, 357-370.
Black, R. A., and J. Hallett, 1998: The mystery of cloud
electrification. American Scientist, 86, 526-534.
Black, R. A., H. B. Bluestein, and M. L. Black, 1994: Unusually strong
vertical motions in a Caribbean hurricane. Mon. Wea. Rev., 122,
2722-2739.
Blanchard, D. O., W. R. Cotton, and J. M. Brown, 1998: Mesoscale
circulation growth under conditions of weak inertial instability.
Mon. Wea. Rev., 126, 118-140.
Bluestein, H. B., 1986: Fronts and jet streaks: A theoretical
perspective. Mesoscale Meteorology and Forecasting, P. S. Ray,
Ed., Amer. Meteor. Soc., 173-215.
Bluestein, H. B., 1993: Synoptic-Dynamic Meteorology in
Midlatitudes. Volume II: Observations and Theory of Weather
Systems. Oxford University Press, 594 pp.
Braun, S. A., and R. A. Houze Jr., 1996: The heat budget of a
midlatitude squall line and implications for potential vorticity
production. J. Atmos. Sci., 53, 1217-1240.
Braun, S. A., and R. A. Houze Jr., 1997: The evolution of the 10-11
June 1985 PRE-STORM squall line: Initiation, development of rear
inflow, and dissipation. Mon. Wea. Rev., 125, 478-504.
Browning, K. A., M. E. Hardman, T. W. Harrold, and C. W. Pardoe, 1973:
The structure of rainbands within a mid-latitude depression. Quart. J. Roy. Meteor. Soc.,
99, 215-231.
Busse, F. H., and W. L. Chen, 1981: On the (nearly) symmetric
instability. J. Atmos. Sci., 38, 877-880.
Byrd, G. P., 1989: A composite analysis of winter season overrunning
precipitation bands over the Southern Plains of the United States.
J. Atmos. Sci., 46, 1119-1132.
Cao, Z., and H.-R. Cho, 1995: Generation of moist potential vorticity
in extratropical cyclones. J. Atmos. Sci., 52, 3263-3281.
Chan, D. S. T., and H.-R. Cho, 1989: Meso-b-scale potential
vorticity anomalies and rainbands. Part I: Adiabatic dynamics of
potential vorticity anomalies. J. Atmos. Sci., 46, 1713-1723.
Chen, C., W.-K. Tao, P.-L. Lin, G. S. Lai, S.-F. Tseng, and
T.-C. C. Wang, 1998: The intensification of the low-level jet during
the development of mesoscale convective systems on a mei-yu front.
Mon. Wea. Rev., 126, 349-371.
Cho, H.-R., and D. Chan, 1991: Meso-b scale potential vorticity
anomalies and rainbands Part II: Moist model simulations. J. Atmos. Sci.,
48, 331-341.
Clough, S. A., and R. A. A. Franks, 1991: The evaporation of frontal
and other stratiform precipitation. Quart. J. Roy. Meteor. Soc., 117, 1057-1080.
Colman, B. R., 1990a: Thunderstorms above frontal surfaces in
environments without positive CAPE. Part I: A climatology. Mon. Wea. Rev.,
118, 1103-1121.
Colman, B. R., 1990b: Thunderstorms above frontal surfaces in
environments without positive CAPE. Part II: Organization and
instability mechanisms. Mon. Wea. Rev., 118, 1123-1144.
Colman, B. R., 1991: Reply. Mon. Wea. Rev., 119, 2514-2515.
Davidson, N. E., K. Kurihara, T. Kato, G. Mills, and K. Puri, 1998:
Dynamics and prediction of a mesoscale extreme rain event in the baiu
front over Kyushu, Japan. Mon. Wea. Rev., 126, 1608-1629.
DeVoir, G. A., 1998: Conditional symmetric instability-Methods of
operational diagnosis and case study of 23-24 February 1994 eastern
Washington/Oregon snowstorm. National Weather Service Western Region
Technical Memorandum 254. [Available from
http://www.wrh.noaa.gov/wrhq/CSITM/webtm.htm.]
Donaldson, N. R., and R. E. Stewart, 1989: On the precipitation
regions within two storms affecting Atlantic Canada.
Atmos.-Ocean, 27, 108-129.
Doswell, C. A. III, 1987: The distinction between large-scale and
mesoscale contribution to severe convection: A case study example.
Wea. Forecasting, 2, 3-16.
Doswell, C. A. III, and E. N. Rasmussen, 1994: The effect of
neglecting the virtual temperature correction on CAPE calculations.
Wea. Forecasting, 9, 625-629.
Doswell, C. A. III, H. E. Brooks, and R. A. Maddox, 1996: Flash flood
forecasting: An ingredients-based methodology. Wea. Forecasting, 11,
560-581.
Doswell, C. A. III, C. Ramis, R. Romero, and S. Alonso, 1998: A
diagnostic study of three heavy precipitation episodes in the western
Mediterranean region. Wea. Forecasting, 13, 102-124.
Ducrocq, V., 1993: Adiabatic and viscous simulations of symmetric
instability: Structure, evolution, and energetics. J. Atmos. Sci., 50,
23-42.
Dudhia, J., 1993: A nonhydrostatic version of the Penn State-NCAR
mesoscale model: Validation tests and simulation of an Atlantic
cyclone and cold front. Mon. Wea. Rev., 121, 1493-1513.
Dunn, L. B., 1988: Vertical motion evaluation of a Colorado snowstorm
from a synoptician's perspective. Wea. Forecasting, 3, 261-272.
Eliassen, A., 1990: Transverse circulations in frontal zones.
Extratropical Cyclones, The Erik Palmén Memorial Volume,
C. W. Newton and E. O. Holopainen, Eds., Amer. Meteor. Soc., 155-165.
Emanuel, K. A., 1979: Inertial instability and mesoscale convective
systems. Part I: Linear theory of inertial instability in rotating
viscous fluids. J. Atmos. Sci., 36, 2425-2449.
Emanuel, K. A., 1980: Forced and free mesoscale motions in the
atmosphere. Collection of Lecture Notes on Dynamics of
Mesometeorological Disturbances. Proceedings of CIMMS Symposium.
Y. K. Sasaki, N. Monji, and S. Bloom, Eds., University of
Oklahoma/NOAA, 191-259.
Emanuel, K. A., 1983a: The Lagrangian parcel dynamics of moist
symmetric stability. J. Atmos. Sci., 40, 2368-2376.
Emanuel, K. A., 1983b: On assessing local conditional symmetric
instability from atmospheric soundings. Mon. Wea. Rev., 111, 2016-2033.
Emanuel, K. A., 1983c: Conditional symmetric instability: A theory
for rainbands within extratropical cyclones. Mesoscale
Meteorology-Theories, Observations and Models, D. K. Lilly and
T. Gal-Chen, Eds., Reidel, 231-245.
Emanuel, K. A., 1985: Frontal circulations in the presence of small
moist symmetric instability. J. Atmos. Sci., 42, 1062-1071.
Emanuel, K. A., 1988: Observational evidence of slantwise convective
adjustment. Mon. Wea. Rev., 116, 1805-1816.
Emanuel, K. A., 1989: The finite-amplitude nature of tropical
cyclogenesis. J. Atmos. Sci., 46, 3431-3456.
Emanuel, K. A., 1990: Appendix to chapter 26b: Notes on the physical
mechanisms of mesoscale precipitation bands. Radar in
Meteorology: Battan Memorial and 40th Anniversary Radar Meteorology
Conference. D. Atlas, Ed., Amer. Meteor. Soc., 473-476.
Emanuel, K. A., 1992: Slantwise circulations in middle latitude
cyclones. Preprints, Fifth Conf. on Mesoscale Processes,
Atlanta, GA, Amer. Meteor. Soc., 233-234.
Emanuel, K. A., 1994: Atmospheric Convection. Oxford University
Press, 580 pp.
Engholm, C. D., E. R. Williams, and R. M. Dole, 1990: Meteorological
and electrical conditions associated with positive cloud-to-ground
lighting. Mon. Wea. Rev., 118, 470-487.
Fischer, C., and F. Lalaurette, 1995a: Meso-b-scale
circulations in realistic fronts. I: Steady basic state. Quart. J. Roy. Meteor. Soc.,
121, 1255-1283.
Fischer, C., and F. Lalaurette, 1995b: Meso-b-scale circulations
in realistic fronts. II: Frontogenetically forced basic states.
Quart. J. Roy. Meteor. Soc., 121, 1285-1321.
Freedman, J., 1995: Winter season positive cloud-to-ground lightning.
M.S. thesis, Dept. of Atmospheric Science, The University at Albany,
State University of New York, 121 pp. [Available from Department of
Earth and Atmospheric Sciences, The University at Albany, State
University of New York, Albany, NY 12222.]
Fritsch, J. M., and G. H. Bryan, 1998: Mesoscale convective systems:
Slab convective overturning of deep moist absolutely unstable layers.
Preprints, 16th Conf. on Weather Analysis and Forecasting,
Phoenix, AZ, Amer. Meteor. Soc., 196-198.
Grumm, R. H., and D. J. Nicosia, 1997: WSR-88D observations of
mesoscale precipitation bands over Pennsylvania.
Natl. Wea. Dig., 21(3), 10-23.
Gu, W., Q. Xu, and R. Wu, 1998: Three-dimensional instability of
nonlinear viscous symmetric circulations. J. Atmos. Sci., 55,
3148-3158.
Gyakum, J. R., 1987: Evolution of a surprise snowfall in the United
States midwest. Mon. Wea. Rev., 115, 2322-2345.
Hobbs, P. V., 1978: Organization and structure of clouds and
precipitation on the mesoscale and microscale in cyclonic storms.
Rev. Geophys. Space Phys., 16, 741-755.
Hoke, J. E., N. A. Phillips, G. J. DiMego, J. J. Tuccillo, and
J. G. Sela, 1989: The Regional Analysis and Forecast System of the
National Meteorological Center. Wea. Forecasting, 4, 323-334.
Holle, R. L., and A. I. Watson, 1996: Lightning during two central
U.S. winter precipitation events. Wea. Forecasting, 11, 599-614.
Holton, J. R., 1992: An Introduction to Dynamic Meteorology.
3rd ed., Academic Press, 511 pp.
Holt, M. W., and A. J. Thorpe, 1991: Localized forcing of slantwise
motion at fronts. Quart. J. Roy. Meteor. Soc., 117, 943-963.
Hoskins, B. J., 1974: The role of potential vorticity in symmetric
stability and instability. Quart. J. Roy. Meteor. Soc., 100, 480-482.
Houze, R. A. Jr., 1993: Cloud Dynamics. Academic Press, 573 pp.
Houze, R. A. Jr., 1997: Stratiform precipitation in regions of
convection: A meteorological paradox? Bull. Amer. Meteor. Soc., 78, 2179-2196.
Houze, R. A. Jr., S. A. Rutledge, M. I. Biggerstaff, and B. F. Smull,
1989: Interpretation of Doppler weather radar displays of midlatitude
mesoscale convective systems. Bull. Amer. Meteor. Soc., 70, 608-619.
Howard, K., and E. Tollerud, 1988: The structure and evolution of
heavy-snow-producing Colorado cyclones. Preprints, Palmén
Memorial Symposium on Extratropical Cyclones, Helsinki, Finland,
Amer. Meteor. Soc., 168-171.
Huo, Z., D.-L. Zhang, J. Gyakum, and A. Staniforth, 1995: A
diagnostic analysis of the Superstorm of March 1993. Mon. Wea. Rev., 123,
1740-1761.
Huschke, R. E., Ed., 1959: Glossary of Meteorology. Amer. Meteor. Soc., 638 pp.
Innocentini, V., and E. dos Santos Caetano Neto, 1992: A numerical
study of the role of humidity in the updraft driven by moist slantwise
convection. J. Atmos. Sci., 49, 1092-1106.
Jascourt, S. D, S. S. Lindstrom, C. J. Seman, and D. D. Houghton,
1988: An observation of banded convective development in the presence
of weak symmetric stability. Mon. Wea. Rev., 116, 175-191.
Jiang, H., and D. J. Raymond, 1995: Simulation of a mature mesoscale
convective system using a nonlinear balance model. J. Atmos. Sci., 52,
161-175.
Johns, R. H., and C. A. Doswell III, 1992: Severe local storms
forecasting. Wea. Forecasting, 7, 588-612.
Jones, S. C., and A. J. Thorpe, 1992: The three-dimensional nature of
`symmetric' instability. Quart. J. Roy. Meteor. Soc., 118, 227-258.
Keyser, D., 1998: On the representation and diagnosis of frontal
circulations in two and three dimensions. The Life Cycles of
Extratropical Cyclones, M. A. Shapiro, Ed., Amer. Meteor. Soc., in
press.
Keyser, D., and L. W. Uccellini, 1987: Regional models: Emerging
research tools for synoptic meteorologists. Bull. Amer. Meteor. Soc., 68,
306-320.
Knight, D. J., and P. V. Hobbs, 1988: The mesoscale and microscale
structure and organization of clouds and precipitation in midlatitude
cyclones. Part XV: A numerical modeling study of frontogenesis and
cold-frontal rainbands. J. Atmos. Sci., 45, 915-930.
Koch, S. E., D. Hamilton, D. Kramer, and A. Langmaid, 1998: Mesoscale
dynamics in the Palm Sunday tornado outbreak. Mon. Wea. Rev., 126,
2031-2060.
Kristjánsson, J. E., and S. Thorsteinsson, 1995: The structure and
evolution of an explosive cyclone near Iceland. Tellus,
47A, 656-670.
Lagouvardos, K., Y. Lemaître, and G. Scialom, 1993: Dynamical
structure of a wide cold-frontal cloudband observed during FRONTS 87.
Quart. J. Roy. Meteor. Soc., 119, 1291-1319.
Lagouvardos, K., and V. Kotroni, 1995: Upper-level frontogenesis:
Two case studies from the FRONTS 87 Experiment. Mon. Wea. Rev., 123,
1197-1206.
Leach, M. J., and J. Kong, 1998: Scale interaction in a California
precipitation event. Preprints, 16th Conf. on Weather Analysis
and Forecasting, Phoenix, AZ, Amer. Meteor. Soc., 270-272.
Lemaître, Y., and J. Testud, 1988: Relevance of conditional symmetric
instability in the interpretation of wide cold frontal rainbands. A
case study: 20 May 1976. Quart. J. Roy. Meteor. Soc., 114, 259-269.
Lemaître, Y., and G. Scialom, 1992: Three-dimensional mesoscale
circulation within a convective postfrontal system. Possible role of
conditional symmetric instability for triggering convective motion.
Quart. J. Roy. Meteor. Soc., 118, 71-99.
Lilly, D. K., 1986: Instabilities. Mesoscale Meteorology and
Forecasting, P. S. Ray, Ed., Amer. Meteor. Soc., 259-271.
Lindstrom, S. S., and T. E. Nordeng, 1992: Parameterized slantwise
convection in a numerical model. Mon. Wea. Rev., 120, 742-756.
Locatelli, J. D., J. E. Martin, and P. V. Hobbs, 1994: A wide
cold-frontal rainband and its relationship to frontal topography.
Quart. J. Roy. Meteor. Soc., 120, 259-275.
Loughe, A. F., C.-C. Lai, and D. Keyser, 1995: A technique for
diagnosing three-dimensional ageostrophic circulations in baroclinic
disturbances on limited-area domains. Mon. Wea. Rev., 123, 1476-1504.
Lussky, G. R., 1989: Heavy rains and flooding in Montana: A case for
operational use of symmetric instability diagnosis. Wea. Forecasting, 4,
186-201.
MacGorman, D. R., and W. D. Rust, 1998: The Electrical Nature of
Storms. Oxford University Press, 422 pp.
Mapes, B. E., 1997: Equilibrium vs. activation control of large-scale
variations of tropical deep convection. The Physics and
Parameterization of Moist Atmospheric Convection, R. K. Smith, Ed.,
Kluwer, 321-358.
Marécal, V., and Y. Lemaître, 1995: Importance of
microphysical processes in the dynamics of a CSI mesoscale frontal
cloud band. Quart. J. Roy. Meteor. Soc., 121, 301-318.
Marks, F. D., Jr., R. A. Houze, Jr., and J. F. Gamache, 1992:
Dual-aircraft investigation of the inner core of Hurricane Norbert.
Part I: Kinematic structure. J. Atmos. Sci., 49, 919-942.
Martin, J. E., 1998: The structure and evolution of a continental
winter cyclone. Part II: Frontal forcing of an extreme snow event.
Mon. Wea. Rev., 126, 329-348.
Martin, J. E., J. D. Locatelli, and P. V. Hobbs, 1992: Organization
and structure of clouds and precipitation on the mid-Atlantic coast
of the United States. Part V: The role of an upper-level front in the
generation of a rainband. J. Atmos. Sci., 49, 1293-1303.
Mass, C. F., and Y.-H. Kuo, 1998: Regional real-time numerical
weather prediction: Current status and future potential. Bull. Amer. Meteor. Soc.,
79, 253-263.
Mathur, M. B., 1983: On the use of lower saturation criteria for
release of latent heat in NWP models. Mon. Wea. Rev., 111, 1882-1885.
Mathur, M. B., 1997: Development of an eye-wall like structure in a
tropical cyclone model simulation. Dyn. Atmos. Oceans,
27, 527-547.
McCann, D. W., 1995: Three-dimensional computations of equivalent
potential vorticity. Wea. Forecasting, 10, 798-802.
McCann, D. W., 1996: Slantwise CAPE-A forecast aid for heavy snow.
Preprints, 15th Conf. on Weather Analysis and Forecasting,
Norfolk, VA, Amer. Meteor. Soc., 120-123.
McCarthy, A. K., 1996: Quantitative evaluation of conditional
symmetric instability as a mesoscale instability mechanism associated
with banded precipitation. M.S. thesis, Dept. of Earth and
Atmospheric Sciences, Saint Louis University, 108 pp. [Available from
Department of Earth and Atmospheric Sciences, Saint Louis University,
3507 Laclede Avenue, St. Louis, MO 63103.]
McNulty, R. P., 1978: On upper tropospheric kinematics and severe
weather occurrence. Mon. Wea. Rev., 106, 662-672.
McNulty, R. P., 1995: Severe and convective weather: A Central
Region forecasting challenge. Wea. Forecasting, 10, 187-202.
Miller, T. L., 1984: The structures and energetics of fully nonlinear
symmetric baroclinic waves. J. Fluid Mech., 142,
343-362.
Miller, T. L., and B. N. Antar, 1986: Viscous nongeostrophic
baroclinic instability. J. Atmos. Sci., 43, 329-338.
Mocko, D. M., and W. R. Cotton, 1995: Evaluation of fractional
cloudiness parameterizations for use in a mesoscale model. J. Atmos. Sci.,
52, 2884-2901.
Molinari, J., and M. Dudek, 1992: Parameterization of convective
precipitation in mesoscale numerical models: A critical review.
Mon. Wea. Rev., 120, 326-344.
Molinari, J., P. K. Moore, V. P. Idone, R. W. Henderson, and
A. B. Saljoughy, 1994: Cloud-to-ground lightning in Hurricane
Andrew. J. Geophys. Res., 99, 16 665-16 676.
Molinari, J., P. Moore, and V. Idone, 1999: Convective structure of
hurricanes as revealed by lightning locations. Mon. Wea. Rev., 127, in
press.
Moore, J. T., and P. D. Blakley, 1988: The role of frontogenetical
forcing and conditional symmetric instability in the midwest snowstorm
of 30-31 January 1982. Mon. Wea. Rev., 116, 2155-2171.
Moore, J. T., and T. E. Lambert, 1993: The use of equivalent potential
vorticity to diagnose regions of conditional symmetric
instability. Wea. Forecasting, 8, 301-308.
Neiman, P. J., M. A. Shapiro, and L. S. Fedor, 1993: The life cycle
of an extratropical marine cyclone. Part II: Mesoscale structure and
diagnostics. Mon. Wea. Rev., 121, 2177-2199.
Nordeng, T. E., 1987: The effect of vertical and slantwise convection
on the simulation of polar lows. Tellus, 39A, 354-375.
Nordeng, T. E., 1993: Parameterization of slantwise convection in
numerical weather prediction models. The Representation of
Cumulus Convection in Numerical Models, Meteor. Monogr., No. 46,
Amer. Meteor. Soc., 195-202.
Orville, R. E., R. W. Henderson, and L. F. Bosart, 1988: Bipole
patterns revealed by lightning locations in mesoscale storm systems.
Geophys. Res. Lett., 15, 129-132.
Parker, D. J., and A. J. Thorpe, 1995: The role of snow sublimation
in frontogenesis. Quart. J. Roy. Meteor. Soc., 121, 763-782.
Parsons, D. B., and P. V. Hobbs, 1983: The mesoscale and microscale
structure and organization of clouds and precipitation in midlatitude
cyclones. XI: Comparisons between observational and theoretical
aspects of rainbands. J. Atmos. Sci., 40, 2377-2397.
Persson, P. O. G., 1995: Simulations of the potential vorticity
structure and budget of FRONTS 87 IOP8. Quart. J. Roy. Meteor. Soc., 121,
1041-1081.
Persson, P. O. G., and T. T. Warner, 1991: Model generation of
spurious gravity waves due to inconsistency of the vertical and
horizontal resolution. Mon. Wea. Rev., 119, 917-935.
Persson, P. O. G., and T. T. Warner, 1993: Nonlinear hydrostatic
conditional symmetric instability: Implications for numerical weather
prediction. Mon. Wea. Rev., 121, 1821-1833.
Persson, P. O. G., and T. T. Warner, 1995: The nonlinear evolution of
idealized, unforced, conditional symmetric instability: A numerical
study. J. Atmos. Sci., 52, 3449-3474.
Ramstrom, W. D., 1990: Wind patterns in New England coastal storms.
B.S. thesis, Dept. of Electrical Engineering and Computer Science,
Massachusetts Institute of Technology, 32 pp. [Available from
Dept. of Electrical Engineering and Computer Science, M.I.T., 77
Massachusetts Avenue, Cambridge, MA 02138.]
Rauber, R. M., M. K. Ramamurthy, and A. Tokay, 1994: Synoptic and
mesoscale structure of a severe freezing rain event: The
St. Valentine's Day ice storm. Wea. Forecasting, 9, 183-208.
Reuter, G. W., and N. Aktary, 1993: A slantwise Showalter index based
on moist symmetric instability: Results for central Alberta.
Atmos.-Ocean, 31, 379-394.
Reuter, G. W., and N. Aktary, 1995: Convective and symmetric
instabilities and their effects on precipitation: Seasonal variations
in central Alberta during 1990 and 1991. Mon. Wea. Rev., 123, 153-162.
Reuter, G. W., and R. Beaubien, 1996: Radar observations of snow
formation in a warm pre-frontal snowband. Atmos.-Ocean,
34, 605-626.
Reuter, G. W., and C. D. Nguyen, 1993: Organization of cloud and
precipitation in an Alberta storm. Atmos. Res., 30,
127-141.
Reuter, G. W., and M. K. Yau, 1990: Observations of slantwise
convective instability in winter cyclones. Mon. Wea. Rev., 118,
447-458.
Reuter, G. W., and M. K. Yau, 1993: Assessment of slantwise convection
in ERICA cyclones. Mon. Wea. Rev., 121, 375-386.
Rivas Soriano, L. J., and E. L. García Díez, 1997: Effect of
ice on the generation of a generalized potential vorticity. J. Atmos. Sci.,
54, 1385-1387.
Rogers, R. R., and M. K. Yau, 1989: A Short Course in Cloud
Physics. 3d ed., Pergamon Press, 293 pp.
Saitoh, S., and H. Tanaka, 1987: Numerical experiments of conditional
symmetric baroclinic instability as a possible cause for frontal
rainband formation Part I. A basic experiment. J. Meteor. Soc. Japan, 65, 675-708.
Saitoh, S., and H. Tanaka, 1988: Numerical experiments of conditional
symmetric baroclinic instability as a possible cause for frontal
rainband formation Part II. Effects of water vapor supply. J. Meteor. Soc. Japan, 66, 39-53.
Sanders, F., 1986: Frontogenesis and symmetric stability in a major
New England snowstorm. Mon. Wea. Rev., 114, 1847-1862.
Sanders, F., and L. F. Bosart, 1985: Mesoscale structure in the
Megalopolitan snowstorm of 11-12 February 1983. Part I:
Frontogenetical forcing and symmetric instability. J. Atmos. Sci., 42,
1050-1061.
Seltzer, M. A., R. E. Passarelli, and K. A. Emanuel, 1985: The
possible role of symmetric instability in the formation of
precipitation bands. J. Atmos. Sci., 42, 2207-2219.
Seman, C. J., 1991: Numerical study of nonlinear convective-symmetric
instability in a rotating baroclinic atmosphere. Ph.D. dissertation,
185 pp. [Available from University of Wisconsin-Madison, Department
of Atmospheric and Oceanic Sciences, Madison, WI 53706.]
Seman, C. J., 1992: On the role of nonlinear convective-symmetric
instability in the evolution of a numerically simulated mesoscale
convective system. Preprints, Fifth Conf. on Mesoscale
Processes, Atlanta, GA, Amer. Meteor. Soc., 282-287.
Seman, C. J., 1994: A numerical study of nonlinear nonhydrostatic
conditional symmetric instability in a convectively unstable
atmosphere. J. Atmos. Sci., 51, 1352-1371.
Shields, M. T., R. M. Rauber, and M. K. Ramamurthy, 1991: Dynamical
forcing and mesoscale organization of precipitation bands in a midwest
winter cyclonic storm. Mon. Wea. Rev., 119, 936-964.
Shutts, G. J., 1990: SCAPE charts from numerical weather prediction
model fields. Mon. Wea. Rev., 118, 2745-2751.
Shutts, G. J., and M. J. P. Cullen, 1987: Parcel stability and its
relation to semigeostrophic theory. J. Atmos. Sci., 44, 1318-1330.
Snook, J. S., 1992: Current techniques for real-time evaluation of
conditional symmetric instability. Wea. Forecasting, 7, 430-439.
Stensrud, D. J., R. A. Maddox, and C. L. Ziegler, 1991: A
sublimation-initiated mesoscale downdraft and its relation to the wind
field below a precipitating anvil cloud. Mon. Wea. Rev., 119,
2124-2139.
Thorpe, A. J., and S. A. Clough, 1991: Mesoscale dynamics of cold
fronts: Structures described by dropsoundings in FRONTS 87. Quart. J. Roy. Meteor. Soc.,
117, 903-941.
Thorpe, A. J., and K. A. Emanuel, 1985: Frontogenesis in the presence
of small stability to slantwise convection. J. Atmos. Sci., 42,
1809-1824.
Thorpe, A. J., and R. Rotunno, 1989: Nonlinear aspects of symmetric
instability. J. Atmos. Sci., 46, 1285-1299.
Thorpe, A. J., B. J. Hoskins, and V. Innocentini, 1989: The parcel
method in a baroclinic atmosphere. J. Atmos. Sci., 46, 1274-1284.
van Mieghem, J. M., 1951: Hydrodynamic instability. Compendium
of Meteorology, T. F. Malone, Ed., Amer. Meteor. Soc., 434-453.
Vonnegut, B., 1994: The atmospheric electricity paradigm. Bull. Amer. Meteor. Soc.,
75, 53-61.
Wallace, J. M., and P. V. Hobbs, 1977: Atmospheric Science: An
Introductory Survey. Academic Press, 467 pp.
Wang, P., Z. Xu, and Z. Pan, 1990: A case study of warm sector
rainbands in north China. Adv. Atmos. Sci., 7,
354-365.
Weisman, R. A., 1996: The Fargo snowstorm of 6-8 January 1989.
Wea. Forecasting, 11, 198-215.
Wiesmueller, J. L., and S. M. Zubrick, 1998: Evaluation and
application of conditional symmetric instability, equivalent potential
vorticity, and frontogenetical forcing in an operational forecasting
environment. Wea. Forecasting, 13, 84-101.
Williams, E., 1991: Comments on ``Thunderstorms above frontal surfaces in
environments without positive CAPE. Part I: A climatology.'' Mon. Wea. Rev.,
119, 2511-2513.
Williams, E., and N. Renno, 1993: An analysis of the conditional
instability of the tropical atmosphere. Mon. Wea. Rev., 121, 21-36.
Wolfsberg, D. G., K. A. Emanuel, and R. E. Passarelli, 1986: Band
formation in a New England winter storm. Mon. Wea. Rev., 114, 1552-1569.
Wood, L. T., and J. W. Nielsen-Gammon, 1994: Synthetic dual-Doppler
analysis of a wintertime rainband. Preprints, Sixth Conf. on
Mesoscale Processes. Portland, OR, Amer. Meteor. Soc., 640-643.
Xu, K.-M., and K. A. Emanuel, 1989: Is the tropical atmosphere
conditionally unstable? Mon. Wea. Rev., 117, 1471-1479.
Xu, Q., 1986a: Conditional symmetric instability and mesoscale
rainbands. Quart. J. Roy. Meteor. Soc., 112, 315-334.
Xu, Q., 1986b: Generalized energetics for linear and nonlinear
symmetric instabilities. J. Atmos. Sci., 43, 972-984.
Xu, Q., 1989a: Extended Sawyer-Eliassen equation for frontal
circulations in the presence of small viscous moist symmetric
stability. J. Atmos. Sci., 46, 2671-2683.
Xu, Q., 1989b: Frontal circulations in the presence of small viscous
moist symmetric stability and weak forcing. Quart. J. Roy. Meteor. Soc., 115,
1325-1353.
Xu, Q., 1992: Formation and evolution of frontal rainbands and
geostrophic potential vorticity anomalies. J. Atmos. Sci., 49, 629-648.
Xu, Q., 1994: Semibalance model-Connection between geostrophic-type
and balanced-type intermediate models. J. Atmos. Sci., 51, 953-970.
Xu, Q., and J. H. E. Clark, 1985: The nature of symmetric instability
and its similarity to convective and inertial instability. J. Atmos. Sci.,
42, 2880-2883.
Zhang, D.-L., and H.-R. Cho, 1992: The development of negative moist
potential vorticity in the stratiform region of a simulated squall
line. Mon. Wea. Rev., 120, 1322-1341.
Zhang, D.-L., and H.-R. Cho, 1995: Three-dimensional simulation of
frontal rainbands and conditional symmetric instability in the
Eady-wave model. Tellus, 47A, 45-61.
Zhao, Q., T. L. Black, and M. E. Baldwin, 1997: Implementation of the
cloud prediction scheme in the Eta model at NCEP. Wea. Forecasting, 12,
697-712.
Ziegler, C. L., and E. N. Rasmussen, 1998: The initiation of moist
convection at the dryline: Forecasting issues from a case study
perspective. Wea. Forecasting, in press.
Table and Figure Captions
Table 1: Definitions of different types of stabilities (Northern
Hemisphere). \dag=At saturation,
[`(q)]e = [`(q)]e*. \ddag=[`(q)]w can be used equivalently
for [`(q)]e.
Figure 1: Number of formal publications that cite
atmospheric ``(conditional) symmetric (in)stability'' or ``slantwise
convection'' as a function of time. Articles are classified
subjectively as to whether they are more
observational/applied-modeling (light shading) or more
theoretical/idealized-modeling (dark shading) studies. References
were compiled through October 1998. Listings of articles were compiled
mainly from literature searches performed by Katherine Day.
Figure 2: An ingredients-based methodology for forecasting
gravitational or slantwise convection.
Figure 3: Rapid Update Cycle (RUC) 9-h forecast verifying
0000 UTC 3 January 1998. Cross section from Dauphin, Manitoba (YDN)
to Minneapolis-St. Paul, Minnesota (MSP): (a) saturated equivalent
potential temperature qe* (solid, every 4 K); (b) equivalent
potential temperature qe (solid, every 4 K).
Figure 4: Stability regimes often observed near
frontal zones. Contours represent typical values often present: Mg
(thick black lines) and qe* (thin grey lines). Based on a
figure originally constructed by Prof. James Moore and Sean Nolan.
Figure 5: (a) Schematic cross section of upscale
convective-symmetric instability in a midlatitude mesoscale
convective system [adapted from Seman (1991, 1992)]. Thick solid line
encloses cloud (shaded). Thin solid lines with arrows represents
direction of circulation. Grey solid line labeled q represents
orientation of typical potential-temperature contour back in the cool
air. Hatching is proportional to precipitation intensity. Moist
gravitational instability is released within the convective line,
remaining moist symmetric instability is released within the trailing
precipitation region; dry adiabatic descent initially occurs within
rear inflow, which may become saturated due to precipitation
evaporating/sublimating into the descending air. (b) Schematic of
downscale convective-symmetric instability as depicted in the
``escalator-elevator'' warm-frontal-ascent model (Neiman et al. 1993,
Fig. 8). Warm southerly airstream (flat, lightly stippled arrows)
rises over the cold easterly polar airstream (tubular dashed arrow).
Mesoconvective ascent (the elevator, solid arrows) and convective
clouds (stippled with white anvils) are shown at regular intervals
between regions of gentler ascent (the escalator).
Figure 6: (a) Case with localized frontogenetical forcing and small
negative MPVg: initial MPVg (contoured every
0.5×10-12 s-4; thick solid, thin solid, and dashed are used for
positive, zero, and negative values, respectively). (b) Case with
localized frontogenetical forcing and small negative MPVg: vertical
velocity at 16.7 h (drawn with power-increasing intervals
±2n×0.5 cm s-1; thick solid, thin solid, and dashed are used
for positive, zero, and negative values, respectively). (c) and (d)
are the same as (a) and (b), respectively, except for case with
localized frontogenetical forcing and large negative MPVg. The
horizontal scale in all panels (from 0.0 to 1.0) is 1000 km. Figures
are from Xu's (1992, Figs. 1b, 2a, 4, and 6a) cases I and II.
Figure 7: Total accumulated 24-h precipitation from
simulations with 80-km horizontal grid-spacing [(a) and (b)] and 40-km
horizontal grid-spacing [(c) and (d)] with Anthes-Kuo convective
parameterization [(a) and (c)] and with both Anthes-Kuo and Nordeng
convective and slantwise parameterizations [(b) and (d)]. Contour
values of 0, 1, 10, 25, 50, 100, and 200 mm shown. (e) Synoptic-scale
analysis of 24-h rainfall ending 1200 UTC 3 December 1982. Contour
values of 12, 25, 50, 75, and 100 mm. (f) Analysis of 24-h rainfall
ending 1200 UTC 3 December 1982 in Arkansas and Missouri. Contour
values of 25, 100, 200, and 300 mm. Figures from Lindstrom and
Nordeng (1992, Figs. 8, 1, and 2, respectively).
Table 1: Definitions of different types of stabilities (Northern
Hemisphere). \dag=At saturation,
[`(q)]e = [`(q)]e*. \ddag=[`(q)]w can be used equivalently
for [`(q)]e.
|
|
|
| | GRAVITATIONAL | SYMMETRIC | INERTIAL |
|
|
|
|
| DRY | absolute instability | symmetric instability | inertial instability
|
| | |
|
| [(d[`(q)])/( dz)] < 0 | [(d[`(q)])/( dz)]|Mg < 0;
[(dMg)/( dx)]|[`(q)] < 0 | [(dMg)/( dx)] < 0
|
| | |
|
| -[(dT)/( dz)] > Gd |
-[(dT)/( dz)]|Mg > Gd|Mg | zg + f < 0
|
| | |
|
| | Mg-[`(q)] relationship |
|
| | |
|
| | PVg < 0 |
|
|
|
|
|
| CONDITIONAL\dag | conditional instability | conditional symmetric
instability | N/A
|
| (CI) | (CSI) |
|
| | |
|
| [(d[`(q)]e*)/( dz)] < 0 |
[(d[`(q)]e*)/( dz)]|Mg < 0;
[(dMg)/( dx)]|[`(q)]e* < 0 |
|
| | |
|
| Gm < -[(dT)/( dz)] < Gd |
Gm|Mg < -[(dT)/( dz)]|Mg < Gd|Mg |
|
| | |
|
| | Mg-[`(q)]e* relationship |
|
| | |
|
| | MPVg* < 0 |
|
|
|
|
|
| POTENTIAL\ddag | potential instability | potential symmetric instability | N/A
|
| (PI) | (PSI) |
|
| | |
|
| [(d[`(q)]e)/( dz)] < 0 |
[(d[`(q)]e)/( dz)]|Mg < 0 ;
[(dMg)/( dx)]|[`(q)]e < 0 |
|
| | |
|
| | Mg-[`(q)]e relationship |
|
| | |
|
| | MPVg < 0 |
|
|
|
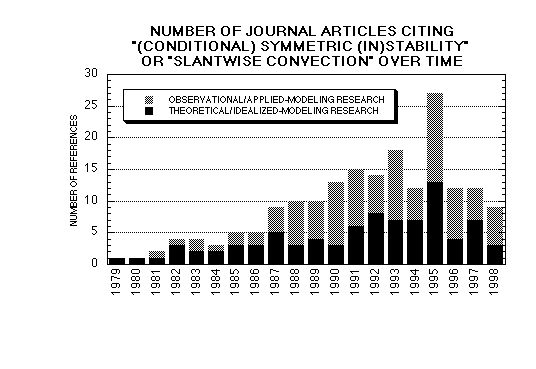
Figure 1: Number of formal publications that cite atmospheric
``(conditional) symmetric (in)stability'' or ``slantwise convection''
as a function of time. Articles are classified subjectively as to
whether they are more observational/applied-modeling (light shading)
or more theoretical/idealized-modeling (dark shading) studies.
References were compiled through October 1998. Listings of articles
were compiled mainly from literature searches performed by Katherine
Day.
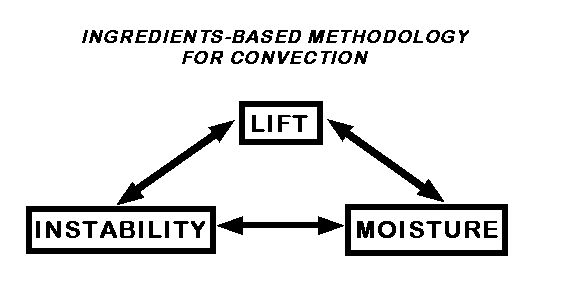
Figure 2: An ingredients-based methodology for forecasting
gravitational or slantwise convection.
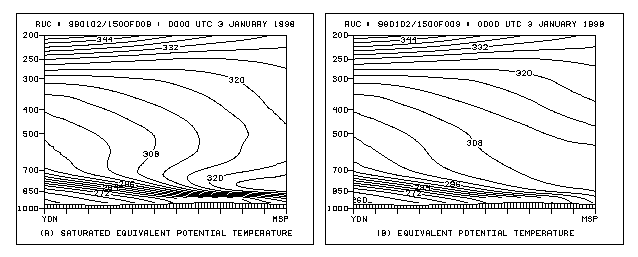
Figure 3: Rapid Update Cycle (RUC) 9-h forecast verifying
0000 UTC 3 January 1998. Cross section from Dauphin, Manitoba
(YDN) to Minneapolis-St. Paul, Minnesota (MSP): (a) saturated
equivalent potential temperature qe* (solid, every 4 K); (b) equivalent
potential temperature qe
(solid, every 4 K).
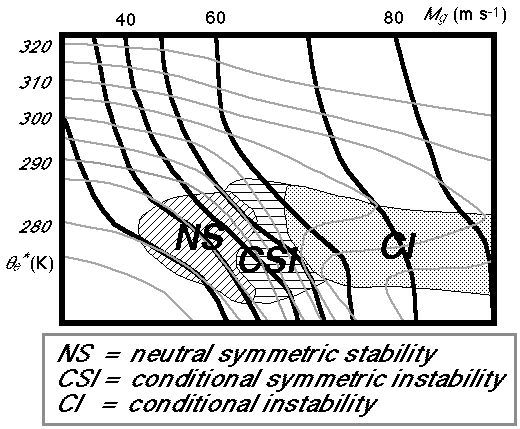
Figure 4:
Stability regimes often observed near frontal zones. Contours
represent typical values often present: Mg
(thick black lines) and qe* (thin grey lines). Based on a figure
originally constructed by Prof. James Moore and Sean
Nolan.
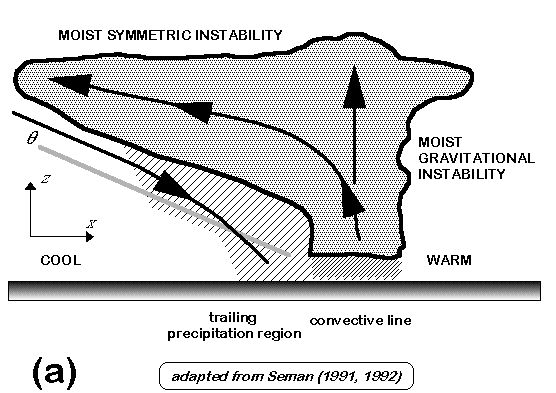
Figure 5: (a) Schematic cross section of upscale convective-symmetric
instability in a midlatitude mesoscale convective system [adapted from
Seman (1991, 1992)]. Thick solid line encloses cloud (shaded). Thin
solid lines with arrows represents direction of circulation. Grey
solid line labeled q represents orientation
of typical potential-temperature contour back in the cool air.
Hatching is proportional to precipitation intensity. Moist
gravitational instability is released within the convective line,
remaining moist symmetric instability is released within the trailing
precipitation region; dry adiabatic descent occurs within rear inflow,
which may become saturated due to precipitation
evaporating/sublimating into the descending air.
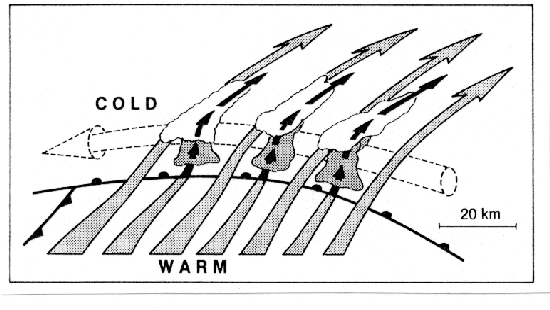
Figure 5: (b) Schematic of downscale
convective-symmetric instability as depicted in the
``escalator-elevator'' warm-frontal-ascent model (Neiman et
al. 1993, Fig. 8). Warm southerly airstream (flat, lightly
stippled arrows) rises over the cold easterly polar airstream (tubular
dashed arrow). Mesoconvective ascent (the elevator, solid arrows) and
convective clouds (stippled with white anvils) are shown at regular
intervals between regions of gentler ascent (the escalator).
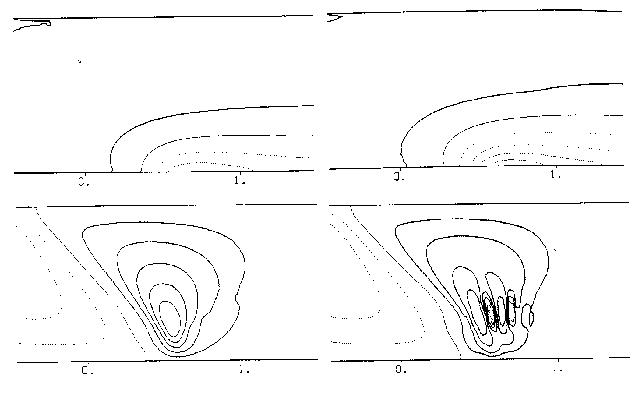
Figure 6: (a) Case with localized
frontogenetical forcing and small negative MPVg:
initial MPVg (contoured every
0.5×10-12 s-4; thick solid, thin
solid, and dashed are used for positive, zero, and negative values,
respectively). (b) Case with localized frontogenetical forcing and
small negative MPVg: vertical velocity at
16.7 h (drawn with power-increasing intervals ±2n×0.5 cm s-1; thick
solid, thin solid, and dashed are used for positive, zero, and
negative values, respectively). (c) and (d) are the same as (a) and
(b), respectively, except for case with localized frontogenetical
forcing and large negative MPVg. The horizontal
scale in all panels (from 0.0 to 1.0) is 1000 km. Figures are
from Xu's (1992, Figs. 1b, 2a, 4, and 6a) cases I and
II.
Figure 7: Total accumulated 24-h
precipitation from simulations with 80-km horizontal grid-spacing [(a)
and (b)] and 40-km horizontal grid-spacing [(c) and (d)] with
Anthes-Kuo convective parameterization [(a) and (c)] and with both
Anthes-Kuo and Nordeng convective and slantwise parameterizations [(b)
and (d)]. Contour values of 0, 1, 10, 25, 50, 100, and 200 mm
shown. (e) Synoptic-scale analysis of 24-h rainfall ending 1200 UTC 3
December 1982. Contour values of 12, 25, 50, 75, and 100 mm. (f)
Analysis of 24-h rainfall ending 1200 UTC 3 December 1982 in
Arkansas and Missouri. Contour values of 25, 100, 200, and 300 mm.
Figures from Lindstrom and Nordeng (1992, Figs. 8, 1, and 2,
respectively).
Footnotes:
1 Also
expressed as qes in
some literature, qe* = qexp(Lqvs/cpT),
where L is the latent heat of vaporization,
qvs is the saturation mixing ratio,
cp is the specific heat of dry air at
constant pressure, and T is the air temperature (e.g., Houze
1993, 54). The saturation equivalent potential temperature is the
equivalent potential temperature the air would have if it were
saturated with water at the same pressure and temperature. When the
air is not saturated, qe* is not conserved, but when the air is
saturated, qe = qe* and, hence, both
are conserved.
2 It was noted during review of this
article that the revised version of the Glossary of Meteorology
will include two definitions for conditional instability
(K. Emanuel 1998, personal communication). The first definition is
the standard one described above [i.e., the environmental lapse rate
lies between the moist and dry adiabatic lapse rates
(Huschke 1959, 125)]. The second definition is a state of
positive convective available potential energy (CAPE). The revision
was deemed necessary because (a) the standard definition does not
necessarily imply a reservoir of potential energy for convection, and
(b) operational usage of the term conditional instability relies more
on measures of parcel buoyancy like CAPE or the lifted index.
3 When the
air motion is not along the Mg surface, the
upper bound of available energy can be estimated by the generalized
energetics of Xu (1986b).
4 It may be that if MSI is small and
negative, then the circulation may approach that of the linear MSI
modes, whereas if the statistical-equilibrium slantwise convection
manifests highly nonlinear motions, the circulations may be strongly
affected or maintained by other processes such as frontogenesis
(Q. Xu 1998, personal communication).
5 Shields
et al. (1991, 951, Figs. 15b and 17b) and Rauber et al. (1994, 197)
also misuse the term CI when what was shown was PI (see
section 3b).
6 The
terminology stratiform precipitation as it pertains to
precipitation in the trailing region of mesoscale convective systems
is discussed by C. Doswell and J. Krácmar
(
http://www.nssl.noaa.gov/ ~ doswell/stratiform/Stratiform__WWW.html) and Houze (1997).
7
Various diagnostic approaches for assessing slantwise convection are
shared at our web site: http://www.nssl.noaa.gov/ ~ schultz/csi.
Return to
The CSI Homepage.
Return to
David Schultz's homepage.




